Infinite knot composed of parallel helices

Question

I am wondering if there is a developed theory of "infinite knots" that could capture this object, and tell me something of its knot properties. Imagine vertical helices in $\mathbb{R}^3$, each discrete $(x,y)$ translations of one helix that spirals around the $z$-axis. Here I show translations just in the $x$-direction:
        
And here in both the $x$- and the $y$-directions:
        
(I tried to ensure that no two helices touch.) Copying the translations to extend the pattern in $(x,y)$, and extending each helix infinitely in the $\pm z$ direction, seems to lead to a structure that is knotted in some sense. Indeed just two helices seem knotted, although I don't know if this "seems" can be made precise.

Can someone point me to literature on definitions and approaches to such infinite knots? Thanks for your ideas and pointers!

Answer 1

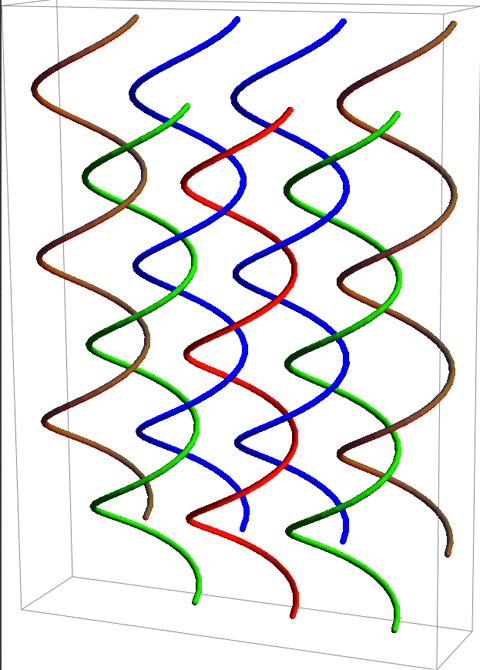
You could consider images such as yours to be a knot in $(S^1)^3$ -- a single-component embedded 1-manifold in the "3-torus" $(S^1)^3$. In your case you get the 3-torus by modding out by your periodicity lattice. In your image there's a rank two part of the lattice in the horizontal plane, and one direction to the lattice that's in a not quite up-down direction, but a little skewed.

Knot theory in $(S^1)^3$ is very similar to knot theory in $S^3$. In fact, there's a way to cook up knots and links in $S^3$ from knots in $(S^1)^3$.

Case 1: you have a knot in $(S^1)^3$ and its homologically trivial. In this case you have a traditional alexander polynomial invariant. This isn't what's going on in your picture, though. The nice thing about this situation is that your knot lifts up to a link in the universal cover. So the components themselves have traditional knot invariants, and you also have various choices of lifts which give choices of links, and they all have invariants of their own.

Case 2: the knot $K$ is homologically non-trivial in $(S^1)^3$. This is what's happening in your picture. In this case, the $H_1$ of the complement of the knot maps onto a free group of rank 2. Moreover, the covering space corresponding to this cover is the complement of a knot in a solid torus. Precisely, the homology of the knot complement is isomorphic to the homology of $(S^1)^3$. $H_1$ of the complement maps onto $H_1((S^1)^3) / H_1(K)$, which is free of rank 2 plus a possible finite cyclic group. Take the map onto the free part.

The covering space corresponding to this map is diffeomorphic to $S^1 \times D^2$, which is an unknot complement. Moreover, since the knot's fundamental class maps to zero in this group, the knot lifts to a knot in this covering space. Putting this all together, associated to a knot in $(S^1)^3$ which is homologically non-trivial you get a $2$-component link in $S^3$ where one component is unknotted. Like case 1, you could make this a many-component link by taking various other lifts of $K$. So there's knot and link invariants associated to these objects, and they're invariants of your original periodic link. In your case, depending on which lifts you take you get components that link each other.

Is there anything more particular you'd like to know about these kinds of invariants? A group of condensed-matter physicists that study knotted light asked me about these kinds of things earlier this summer.

Answer 2

These are the unlink.

If you take any $z$ cross-section, you see a discrete collection of points in the plane, say countably many. So you have a map $\varphi: \mathbb{N}\times \mathbb{R} \to \mathbb{R}^3$ such that $\varphi(n, z) = (f_1(n,z),f_2(n,z), z)$. Claim: one may find a smoothly varying family of diffeomorphisms $\phi_z: \mathbb{R}^2\to \mathbb{R}^2$ such that $\phi_z((n,0))=(f_1(n,z),f_2(n,z))$. Then we obtain a diffeomorphism $\Phi: \mathbb{R}^3\to\mathbb{R}^3$ defined by $\Phi(x,y,z)=\phi_z(x,y)$, which sends the infinite unlink $\mathbb{N}\times \{0\}\times \mathbb{R}$ to the given braid. Since $Diff_+(\mathbb{R}^3)$ is connected, this diffeo. may be achieved by ambient isotopy.

Here's a proof of the claim. First, let's see how to extend a single point moving smoothly in the plane to a family of diffeos. Let $\phi^1_z(x,y)=(f_1(0,z)+x,f_2(0,z)+y)$. Then $\phi^1_z(0,0)=(f_1(0,z),f_2(0,z))$. Thus, we can "straighten" a single strand by composing with this diffeomorphism.

Assume we have "straightened" $n$ strands. To straighten the $n+1$st strand, we choose the same diffeo. above tailored to this strand. This diffeo. is tangent to a 1-parameter family of vector fields in the plane. We may modify these vector fields by a bump function to be zero in a neighborhood of the first $n$ points which avoids the $n+1$st point. Then integrate this vector field to get a family of diffeomorphisms which fixes the first $n$ points. One may be a bit more careful with this induction to make sure that as $n\to \infty$, this family of diffeomorphisms is eventually constant on compact subsets of the plane. Then one obtains in the limit the desired 1-parameter family of diffeos. which straightens the link.