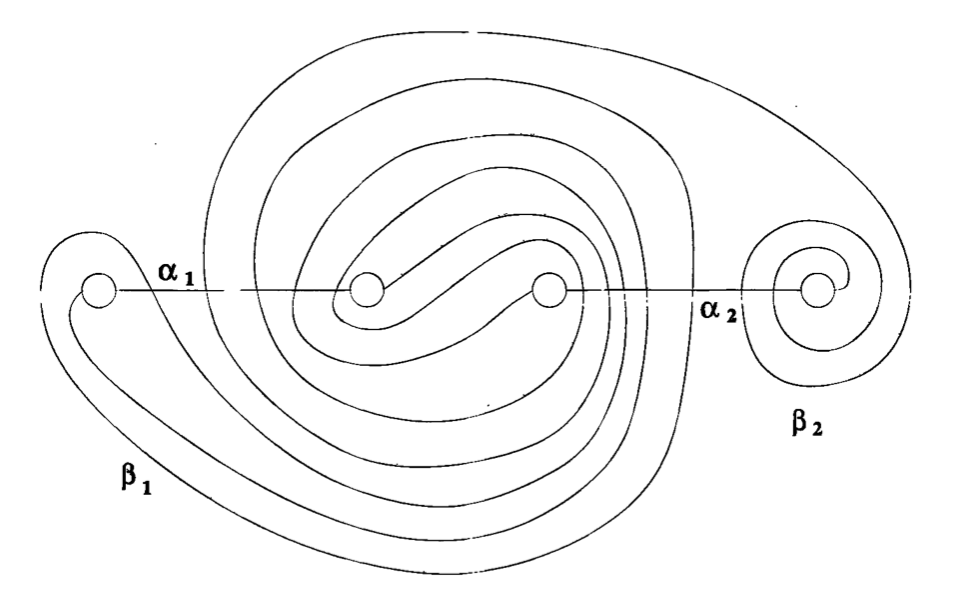
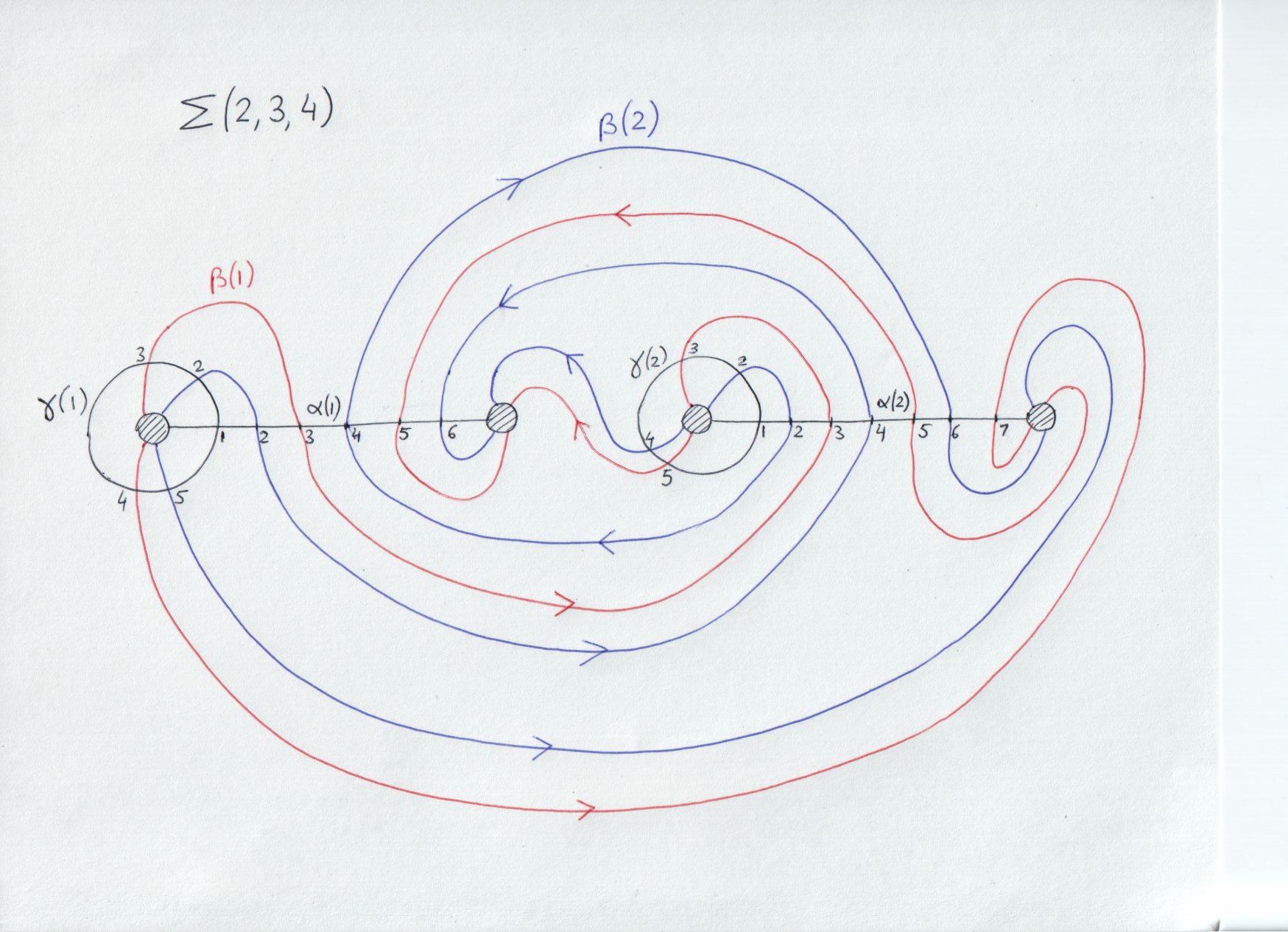
The genus $g$ handlebodies are building blocks of $3$-manifolds. They are constructed from $3$-ball $B^3$ by adding $g$-copies of $1$-handles $B^2 \times B^1$. Their boundaries are homeomorphic to the genus $g$ surface $\Sigma_g$.
It turns out that any closed orientable $3$-manifold $Y$ can be obtained by gluing together two handlebodies $H_1$ and $H_2$ (such a decomposition is called Heegaard splitting):
- $Y= H_1 \cup H_2$,
- $\partial H_1 = \partial H_2 = \Sigma_g$.
The basic examples of such $3$-manifolds are
- $S^3$,
- $S^1 \times S^2$,
- $S^1 \times S^1 \times S^1$,
- Lens spaces $L(p,q) = S^3 / \mathbb Z_p$,
- Brieskorn spheres $\Sigma(p,q,r) = \{ x^p + y^q +z^r = 0 \} \cap S^5 \subset \mathbb C^3$.
There are many references for Heegaard splittings of the first four of these examples, for example chapter 1 of Saveliev's book: Lectures on the Topology of 3-Manifolds.
How about the Brieskorn spheres? Is there an easy way to think about their Heegaard splittings? How can we draw them? Is there any good reference for this?