Is the point giving the width in strictly convex surface a cut point?

Question

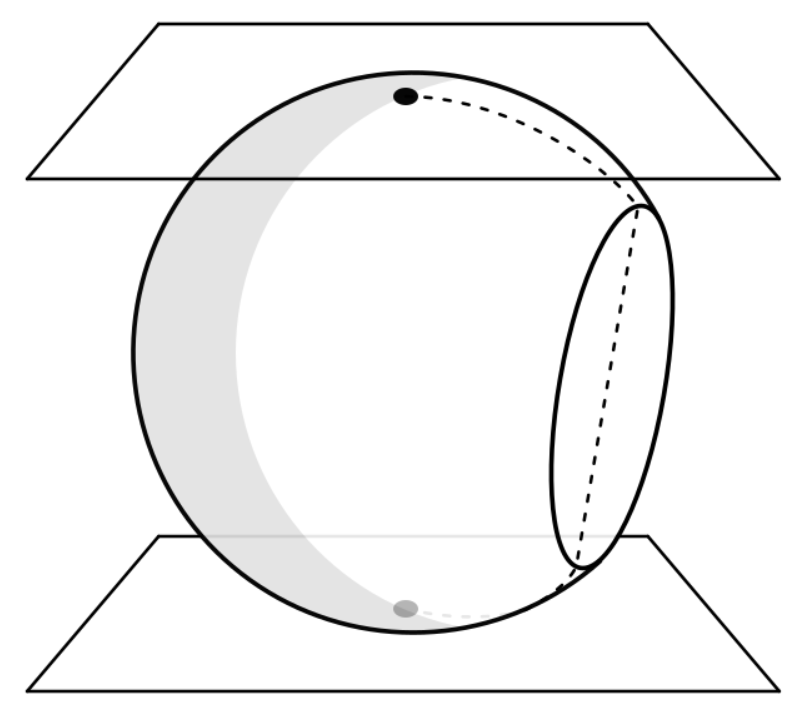
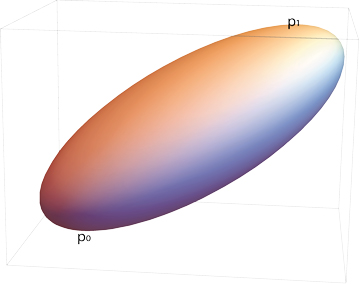
Assume that $\Sigma$ is a stricly convex surface in $\mathbb{E}^3$ homeomorphic to a sphere. Further, assume that $p_0,\ p_1\in \Sigma$ are intersection points with planes $z=0,\ z=1$ and the surface $\Sigma$ is between the two planes. Then $p_1$ is a cut point of $p_0$ ? (I do not know whether or not this is true)

Definition : Consider a intrinsic metric on $\Sigma$, a length of simple path. Then $p_1$ is not a cut point of $p_0$ if there is unique shortest path from $p_0$ to any point sufficiently close to $p_1$.

Answer 1

No.

It is clear that this is true for a sphere, but cutting of a spherical cap from the sphere leaves the intersection points with the planes the same, but now there is a unique shortest path through this new flat area.

$\qquad\qquad\qquad\qquad\quad$

Of course you can make this object strictly convex, differentiable etc. by smoothing out the cut.

Answer 2

Intuitively, it seems that there is no reason that $p_1$ in a tilted ellipsoid is a cut point of $p_0$. Because the orientation of the ellipsoid is a global issue (i.e., the location of $p_0$ and $p_1$ change with the tilt), whereas cut-point-ness is intrinsic.

---

---

In this symmetric example, perhaps there are a pair of equal-length geodesics connecting $p_0$ to $p_1$, but if I arranged $\Sigma$ to be asymmetric with respect to the coordinate axes, there would be a unique shortest geodesic connecting those two $z=0,1$ tangency points.