No.
It is clear that this is true for a sphere, but cutting of a spherical cap from the sphere leaves the intersection points with the planes the same, but now there is a unique shortest path through this new flat area.
$\qquad\qquad\qquad\qquad\quad$ 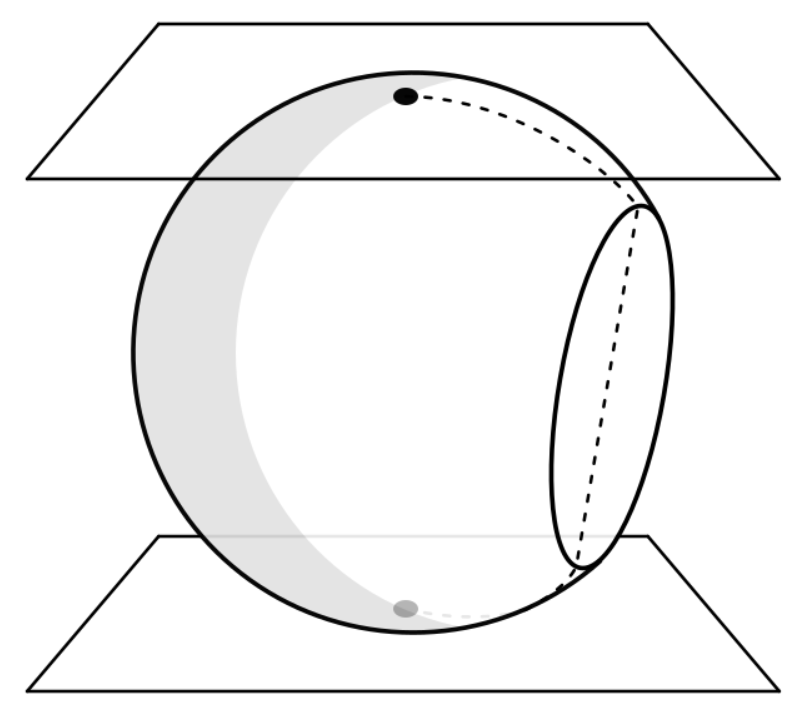
Of course you can make thethis object strictly convex, differentiable etc. by smoothing out the cut.
