Per the title, what are some of the oldest books on series out there with unsolved exercises? Maybe there are some hidden gems from before the 20th century out there.
$\begingroup$
$\endgroup$
3
asked Aug 16, 2019 at 23:50
Squid with Black Bean SauceSquid with Black Bean Sauce
1,5321 gold badge18 silver badges38 bronze badges
-
3$\begingroup$ This question is probably better suited for MathStackExchange. Nevertheless, if you work all the exercises in Whittaker and Watson, you will have seen most of the gems. They are not hidden. The best mathematicians do not forget history, by any means. $\endgroup$– paul garrettCommented Aug 16, 2019 at 23:52
-
3$\begingroup$ Also, I see from your other questions that you are asking several nearly identical questions... best to try to organize these, and to ask them on MathStackExchange, etc. And, again, it's not really that anything "got lost". Perhaps a shallow version of contemporary science/math operates in a way that systematically ignores anything not on-line and less than 10 years old, but serious mathematicians do not take that approach. So there is a problem with your (implicit) premise, too. $\endgroup$– paul garrettCommented Aug 16, 2019 at 23:56
-
2$\begingroup$ Part of a series: mathoverflow.net/questions/327697/… and mathoverflow.net/questions/337524/… and mathoverflow.net/questions/324717/… and mathoverflow.net/questions/326104/… and mathoverflow.net/questions/313967/… ... $\endgroup$– Gerry MyersonCommented Aug 19, 2019 at 23:22
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
Two classics from a century ago, with a great variety of unsolved exercises:
Theorie und Anwendung der unendlichen Reihen, by Konrad Knopp (1922) --- English translation
An Introduction to the Theory of Infinite Series by Thomas Bromwich (1908)
An older but more elementary text is Introduction to Infinite Series by William Osgood (1897)
An exercise from Knopp:
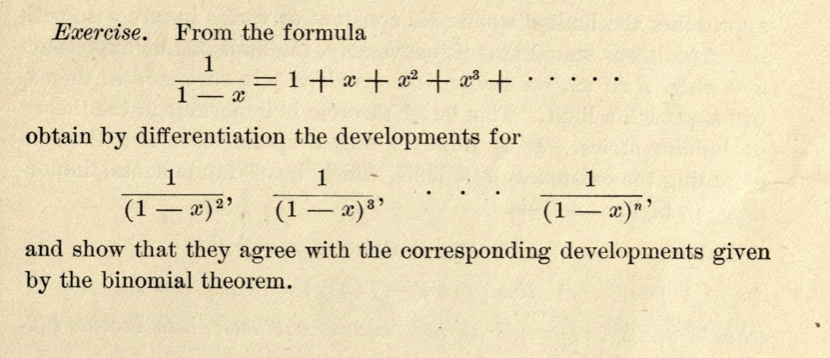
An exercise from Bromwich:
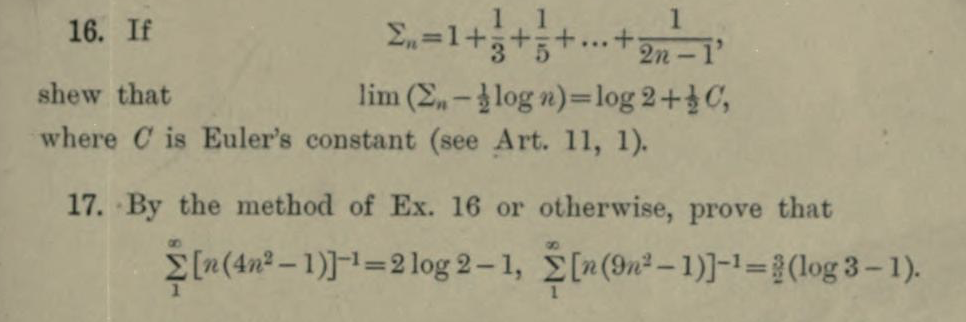
An exercise from Osgood:
answered Aug 20, 2019 at 8:51