6
$\begingroup$

Let $M$ be a smooth closed positively curved surface in Euclidean 3-space, $T$ be a geodesic triangulation of $M$, and $E$ be the edge graph of the convex hull of vertices of $T$.

It is easy to see that $T$ may not always be isomorphic to $E$, because $E$ is determined by the vertices of $T$, while a set of points in $M$ may form vertices of different triangulations of $M$. For instance, one might take any pairs of adjacent acute triangles in $T$ and replace their common edge with a geodesic connecting the vertices of these triangles not on that edge.

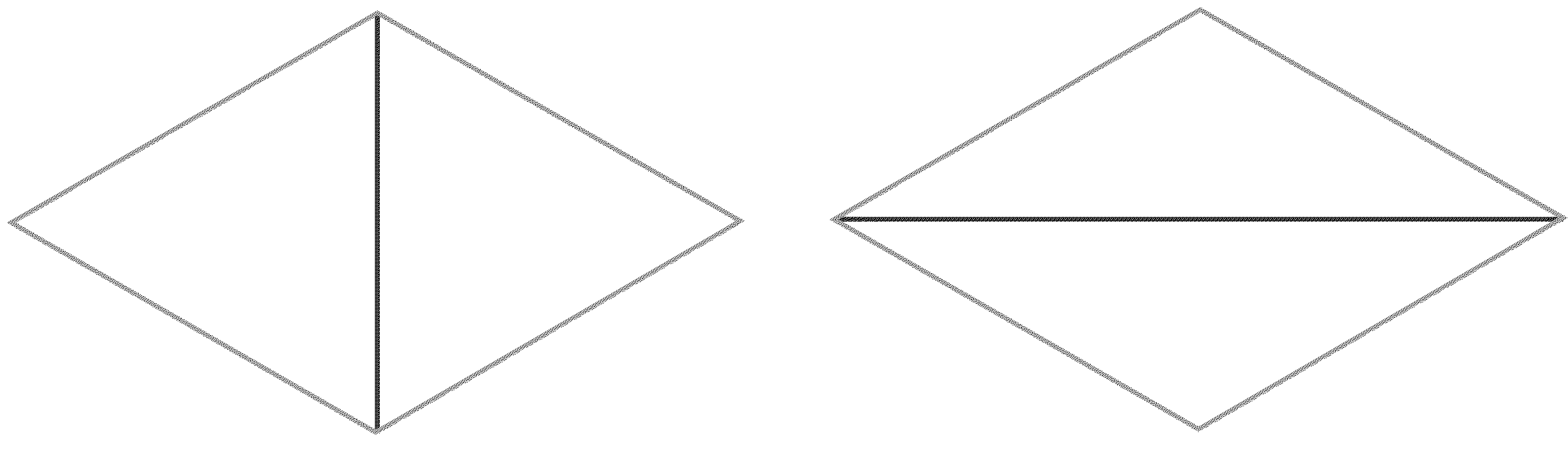
Question: Are there some conditions which one may impose on $T$ as a subset of $M$ (e.g., involving edge lengths, angles, or curvatures of $M$) to ensure that $T$ is isomorphic to $E$?

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ It is unknown even if $M$ is the surface of convex hull of vertexes of $T$. In other words you look at the PL metric with nonnegative curvature on the sphere and want find where it will folds once you apply Alexandrov's embedding theorem --- there is no way to see it before applying the theorem. $\endgroup$
    – Anton Petrunin
    Commented May 12, 2018 at 17:36
  • $\begingroup$ A promising candidate condition would be that the $T$ coincide with points, in which both principal curvatures attain a local maximum. Those points would be the natural smooth generalization of the corners of convex polyhedra. Further candidate points would be those where either both principal curvature attain local extrema or, maybe also other points on ridges $\endgroup$
    – Manfred Weis
    Commented May 13, 2018 at 14:47

1 Answer 1

Reset to default
-1
$\begingroup$

Both your pics represent a triangulation of the patch.

If the vertices are situated on a sphere, the overlay of both triangulations represents the face set of a simplex.

Obviously the longer diagonal is the one which cuts deeper into the ball and thus is concave. Therefore that one is to be excluded, when looking for the convex hull.

Only in case of a regular square patch both diagonals bear a flat dihedral angle, and thus could be used in your searched for triangulation interchangeably. - But the convex hull then would NOT be a triangulation, rather it would use that very square!

Btw. the same holds true, whenever the 2 diagonals are of the same size and the mutual intersection point hits both diagonals in exactly the same ratio. Then the 4 vertices would represent a flat tetragon.

--- rk

Improve this answer
$\endgroup$
3
  • $\begingroup$ I disagree that the longer diagonal lies below the shorter diagonal. Take a kite inscribed in a circle and push its smaller diagonal towards the center of the sphere. $\endgroup$
    – Ivan Izmestiev
    Commented May 16, 2018 at 14:12
  • $\begingroup$ @IvanIzmestiev: if the vertices are on the sphere, then the larger distance always will be deeper to the center than the shorter distance, for sure! $\endgroup$
    – Dr. Richard Klitzing
    Commented May 16, 2018 at 15:31
  • 1
    $\begingroup$ If by "deeper" you mean closer to the center of the sphere, then I agree. But if you take the half-line from the center of the sphere that intersects both segments, then it can happen that it intersects the shorter of the two segments first. $\endgroup$
    – Ivan Izmestiev
    Commented May 17, 2018 at 16:13

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .