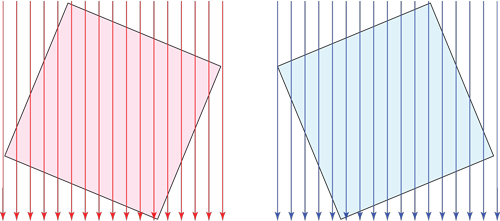
Consider a closed smooth bounded curve enclosing a region $S$ in the XY-plane $\mathbb{R} ^2$.
We define the function $f(x)$, where $x$ is a point on the $x$ axis, as the length of the intersection of the line paralell to the $y$-axis which goes through $x$, with the set $S$, so it can have multiple components and we know the total length.
Suppose we know the same function for some coordiante systems rotated relative to the xy system. Can we reconstruct $S$ using only this data? How many coordinate systems do we need?