In the knot table, it is well-known that 8_19 is (3,4)-torus knot. But, it is not clear to me. How can i change 8_19 to (3,4)-torus knot K(3,4)? Moreover, it is well-known that braids of two equivalent knots are related by Markov moves. How can i change a braid presentation of 8_19 to a braid presentation of K(3,4) by using Markov moves?
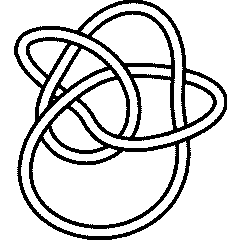
This is an easy exercise, once you know about braid closures. For a reference, see Chapter III of "Knots, links, braids, and 3-manifolds" by Prasolov and Sossinsky.
Here is a sketch of what you need to do.
Place a point $0$ in the central triangle of the figure. Orient $K$ so it winds $+3$ times about $0$. Draw a straight ray $R$ going directly down from $0$. Rotate $R$ around $0$ in the anti-clockwise direction. Record the crossings as $R$ passes them. The orientation allows us to record each crossing as $\sigma_1^{\pm 1}$ or $\sigma_2^{\pm 1}$. (In fact, in this example all crossings are positive.)
Write out the word you get. A single Markov III move (in the braid group, $\sigma_2 \sigma_1 \sigma_2 = \sigma_1 \sigma_2 \sigma_1$) transforms your word into $(\sigma_1 \sigma_2)^4$: the closure of this word is the $(3,4)$ torus knot.
Here is an explicit move that transforms the diagram shown into the standard projection of the torus knot. Consider the upper triangle in the diagram, and label the three crossings at its vertices as 1, 2, 3 from left to right. Now take the (1,2) edge of the triangle and drag this to the right across the vertex 3. The result is the standard projection of a torus knot, where the crossing go "over, over, under, under, over, over, etc." as one proceeds around the knot. (Pictures would describe this isotopy better than words.)
Added later: Different knot tables seem to show different projections of $8_{19}$. The figure that Ryan added to the original question is the $8_{19}$ knot as shown in what the Knot Atlas webpage calls "The Rolfsen Knot Table", but Rolfsen's book actually has a different projection where it takes two moves of the sort I described to get to the standard projection of a torus knot.
