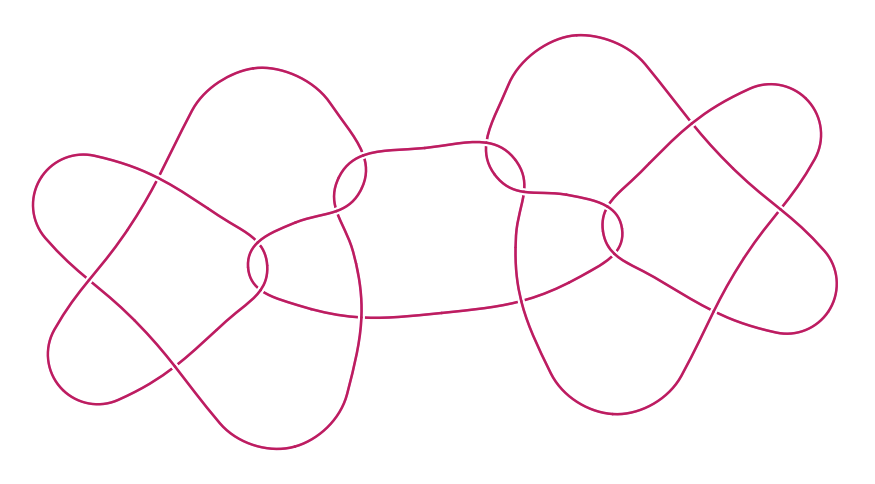
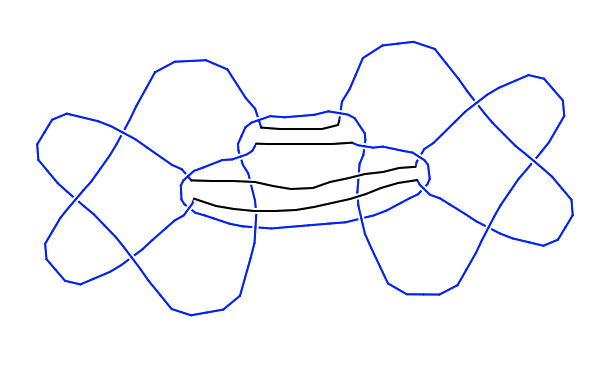
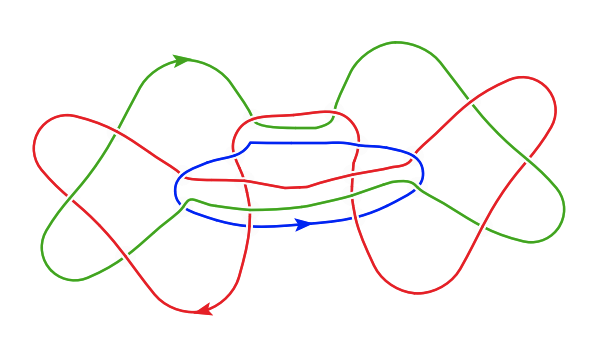
Let $K$ be a knot in $S^3$. It is well-known that the knot $K \# -\overline{K}$ is always ribbon.
The following picture describes the connected sum of the left-handed torus knot $T(3,4)$ and the right-handed torus knot $T(3,4)$. In Rolfsen's notation, $T(3,4) = 8_{19}$, for several descriptions see [1] and [2].
I would like to find the ribbon move(s) for this composite knot but I could not elaborate.
Is there an easy way to see this or any trick to figure out the necessary ribbon moves?
If you want to find a set of ribbon moves, then you need to end up with an unlink with several components; therefore, you need to attack crossings!
This is your diagram:
Then I add two bands along twists of given torus knots:
Next, we have a link with three components:
If you apply Reidemeister moves, you may eventually find an unlink with three components:
There is no specific trick for any torus knot (my opinion) but you can control the number of additional ribbon moves.
Assume that $p$ and $q$ are relatively coprime integers with $p < q$. Then for the corresponding ribbon knot $T(p,q) \# \overline{T(p,q)} $, the number of ribbon bands is $p-1$.
As this composite knot is an example of a symmetric union of knots I suggest that you read my paper "The search for nonsymmetric ribbon knots" (see for instance Figures 1 and 4 and the explanation in the text).
