The more recent paper I've found about this problem is by Michael Aschbacher in 2013:
Overgroup lattices in finite groups of Lie type containing
a parabolic
His introduction is a short survey of the last advanced, he recall Palfy–Pudlak theorem and question, and focus on the following John Shareshian's conjecture. His paper is a first step for a proof of this conjecture:
Let $B_n$ be the subgroups lattice of the cyclic group $C_m$ with $m=p_1p_2 \dots p_n$ square free and $p_i$ prime number.
Let $\mathcal{L}$ be a finite lattice and $\mathcal{L}'$ the poset $\mathcal{L}-\{l,g \}$ with $l$ and $g$ the least and greatest elements of $\mathcal{L}$.
Shareshian's Conjecture: If $\mathcal{L}'$ is a disconnected graph with connected components $(B'_{n_i})_{i=1, \dots , k}$ and $n_i \ge 3$, then $\mathcal{L}$ is not an intermediate subgroups lattice.
The smallest lattice $\mathcal{L}$ coming from this conjecture is the following:
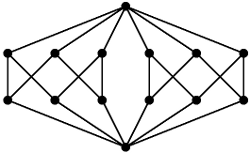
with $k=2$, $n_1=n_2=3$

