3
$\begingroup$

Is there a supersolvable group $G$ with the lattice of all its normal subgroups, order-isommorphic to the 18-element lattice of down-sets of this poset:

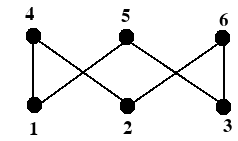
enter image description here

?

It has been proved that not every finite distributive lattice can be represented as the normal subgroup lattice of a supersolvable group.

comments:

By this paper,there is not any supersolvable group order isomorphic to this lattice.

Improve this question
$\endgroup$
2
  • $\begingroup$ i.sstatic.net/tGW06.png $\endgroup$
    – Minimus Heximus
    Commented Oct 24, 2015 at 15:28
  • $\begingroup$ Not sure if this helps, and this comment is belated, but anyway - the lattice is the quotient of the free distributive lattice on three generators $x_1,x_2,x_3$ by $x_1\land x_2\land x_3=\bot$ and $x_1\lor x_2\lor x_3=\top$, so, if the free one is realizable via some subgroups $H_1$, $H_2$, $H_3$, the needed realization will be via normal subgroups of $\langle H_1,H_2,H_3\rangle/H_1\cap H_2\cap H_3$ $\endgroup$
    – მამუკა ჯიბლაძე
    Commented Jun 3, 2017 at 8:58

1 Answer 1

Reset to default
1
$\begingroup$

Based on a some similar argument due to Roland Schmidt, I think you can take $6$ pairwise different primes $p_1$,$p_2$,$p_3$,$p_4$,$p_5$,$p_6$ representing $1$,$2$,$3$,$4$,$5$,$6$, in the diagram, satisfying the conditions $p_4$ divides $p_1-1$ and $p_2-1$, ... such that if $P_1$,$P_2$,$P_3$,$P_4$,$P_5$,$P_6$ are groups of orders $p_1$, $p_2$, $p_3$, $p_4$, $p_5$, $p_6$, respectively, then the semidirect product $G$ of the cyclic group $C=P_1 \times P_2 \times P_3$ by the cyclic group $D=P_4 \times P_5 \times P_6$ exists in which the operations of $P_4$ are nontrivial on $P_1$ and $P_2$ but trivial on $P_3$, $P_5$ nontrivial on $P_1$ and $P_3$ but trivial on $P_2$, and $P_6$ nontrivial on $P_2$ and $P_3$ but trivial on $P_1$. Then it can be proved that one gets the lattice of normal subgroups in the diagram.

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ So more specifically one may take the group with generators $x_3,x_5,x_7,x_{31},x_{43},x_{71}$ and relations $x_p^p=1$, $p\in\{3,5,7,31,43,71\}$, $[x_3,x_{31}]=x_{31}^4$, $[x_5,x_{31}]=x_{31}$, $[x_3,x_{43}]=x_{43}^5$, $[x_7,x_{43}]=x_{43}^3$, $[x_5,x_{71}]=x_{71}^4$, $[x_7,x_{71}]=x_{71}^{19}$, and all remaining commutators trivial. But I do not see straight away whether the lattice of normal subgroups is as required. $\endgroup$
    – მამუკა ჯიბლაძე
    Commented Jun 3, 2017 at 9:49
  • $\begingroup$ you can add it as a more detailed answer. Then I will remove my answer. Because I have not tested the details @მამუკაჯიბლაძე $\endgroup$
    – Minimus Heximus
    Commented Jun 3, 2017 at 13:56
  • $\begingroup$ I don't think it would be right, the idea is yours, and moreover as I said I have not thought how to prove that the lattice is the one needed. Specifically I do not quite see that the indecomposable normal subgroups upstairs are precisely $\langle x_3,x_{31},x_{43}\rangle$, $\langle x_5,x_{31},x_{71}\rangle$ and $\langle x_7,x_{43},x_{71}\rangle$, (the ones downstairs are clearly $\langle x_{31}\rangle$, $\langle x_{43}\rangle$ and $\langle x_{71}\rangle$). They are all indecomposable, but why are there no other indecomposable normal subgroups? $\endgroup$
    – მამუკა ჯიბლაძე
    Commented Jun 3, 2017 at 16:14
  • $\begingroup$ to prove if the lattices are the same one has just to find all subgroups of $G$ (and know which one contains the other). Maybe GAP can find them. $\endgroup$
    – Minimus Heximus
    Commented Jun 3, 2017 at 18:02
  • 1
    $\begingroup$ In fact it now occurred to me - this is the semidirect product $C_{31\times43\times71}\rtimes C_{3\times5\times7}$, with the generator $a:=x_3x_5x_7\in C_{3\times5\times7}$ acting on the generator $b:=x_{31}x_{43}x_{71}\in C_{31\times43\times71}$ via $b\mapsto x_{31}^{10}x_{43}^{24}x_{71}^{100}=x_{31}^{10}x_{43}^{24}x_{71}^{29}=b^{8194}$ $\endgroup$
    – მამუკა ჯიბლაძე
    Commented Jun 3, 2017 at 18:44

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .