1
$\begingroup$

This question might be more suitable for http://math.stackexchange.com. I'm not sure about the differences between that website and this website (http://mathoverflow.net), so I'll try it here first.

All the zeros of the Riemann Zeta function on the complex plain are located either on the $[a+0i]$ line (known as trivial zeros), or between the $[0+ti]$ line and the $[1+ti]$ line (known as non-trivial zeros):

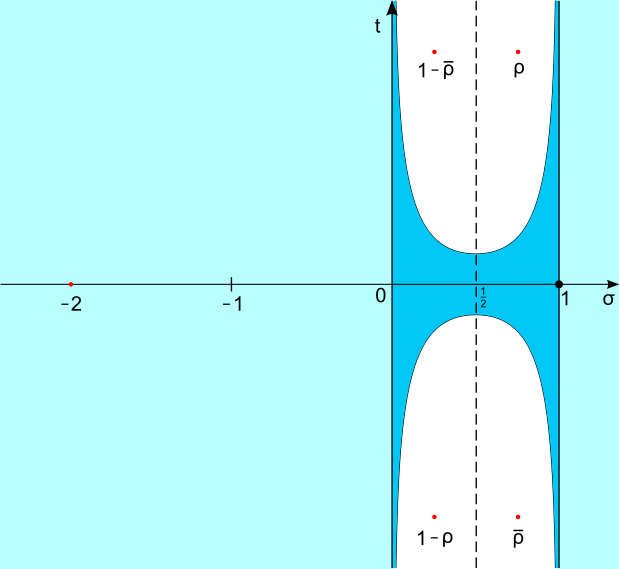
enter image description here

My question is with regards to the following facts:

I assume that none of them has been refuted, but has any of them been proved?

  • There is a finite number of non-trivial zeros that are not located on the $[\frac{1}{2}+ti]$ line.

  • There is a line in the form $[a+ti]$ with $0<a<1$, on which there is a finite number of zeros.

Thanks

Improve this question
$\endgroup$
6
  • $\begingroup$ Any positive or negative answer to your questions would resolve the Riemann hypothesis, so no, this has not been proved. $\endgroup$
    – Per Alexandersson
    Commented Mar 26, 2014 at 11:35
  • $\begingroup$ @Per Alexandersson: Thanks. First of all, a negative answer would simply "leave it as is" (would not resolve RH), but that's just a logical misinterpretation of yours I suppose. Second, I can understand why proving the first fact might resolve RH (although it still requires an explanation). But why would proving the second fact resolve RH? If there is one such line (or even an infinite number of such lines), there can still be non-trivial zeros on other lines. $\endgroup$
    – barak manos
    Commented Mar 26, 2014 at 11:40
  • $\begingroup$ See also mathoverflow.net/questions/161442/…. $\endgroup$
    – Dietrich Burde
    Commented Mar 26, 2014 at 12:22
  • $\begingroup$ @barakmanos: Ah, yes, 0 is a finite number also. That is what made me confused. So, your question is: Can there be an infinite number of counter-examples to RH, and "can there be an infinite number of counter-examples to RH on a vertical line". This formulation (I think) is much more clear. $\endgroup$
    – Per Alexandersson
    Commented Mar 26, 2014 at 12:55
  • $\begingroup$ @Per Alexandersson: What I meant is, that a negative answer to the question "has any of them been proved?" would not resolve anything ("no, none of them has been proved" is equivalent to "everything remains as is"). $\endgroup$
    – barak manos
    Commented Mar 26, 2014 at 13:06

1 Answer 1

Reset to default
8
$\begingroup$

For the second question, the number of zeros being countable, there exist uncountably many vertical lines without any zeros...

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thank you. Doesn't that mean that there is a finite number of vertical lines with zeros (since the number of real values between 0 and 1 is "equally" uncountable)? Also, is there at least one specific line without any zeros whose Real value is known? $\endgroup$
    – barak manos
    Commented Mar 26, 2014 at 13:03
  • $\begingroup$ For the first, no, as far as I know there could be a zero with real part given by any rational number between 1/2 and 1; for the second, also no, there is no explicit vertical line without zeros that is currently known (again, to my knowledge). $\endgroup$
    – Denis Chaperon de Lauzières
    Commented Mar 26, 2014 at 14:44
  • $\begingroup$ OK, thank you very much for your answer. $\endgroup$
    – barak manos
    Commented Mar 26, 2014 at 15:35

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .