6
$\begingroup$

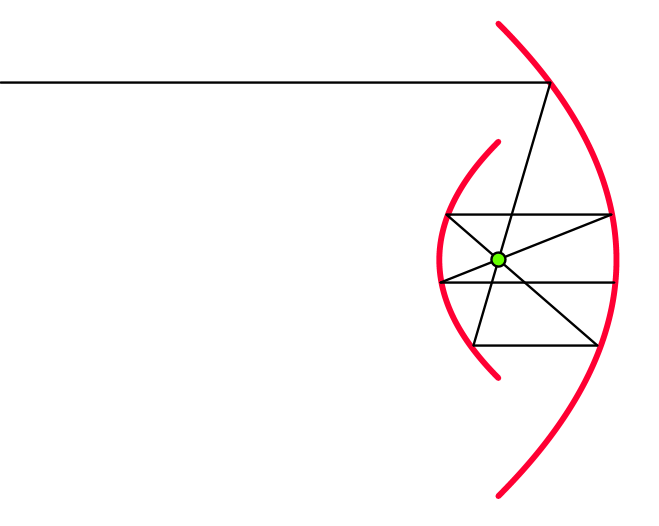
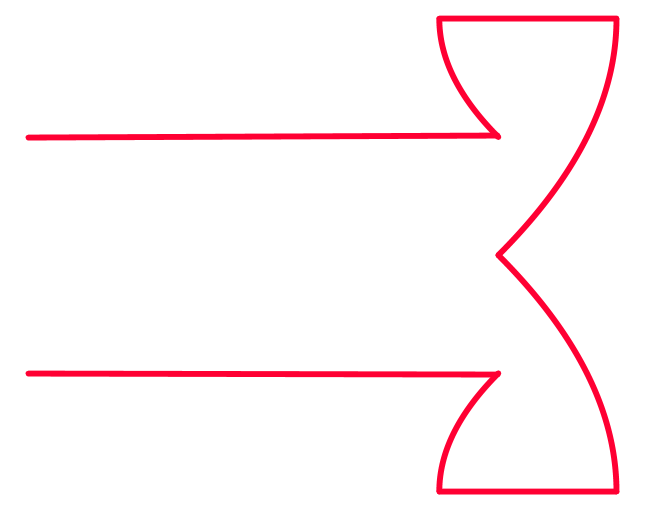
Is there a curve $C$ that connects $(0,1)$ to $(a,0)$ for some $a>0$, and, when reflected to $C'$ in the $x$-axis, the shape $S=C \cup C'$ has the property that each horizontal light ray entering $S$ from the left reflects off of $S$ (interpreted as a perfect mirror) in such a way that it never emerges, i.e. it never again crosses $x=0$?

For example, a straight line $C$ fails to be such a curve:
     BlackHoleNot

Improve this question
$\endgroup$
9
  • $\begingroup$ I think that would violated Liouville's theorem (this one: en.wikipedia.org/wiki/Liouville%27s_theorem_%28Hamiltonian%29). $\endgroup$
    – Yoav Kallus
    Commented Aug 13, 2013 at 20:52
  • $\begingroup$ @Joseph: Are you looking for a curve which causes an incoming light ray to reflect infinitely many times on it without crossing the line $x=0$? Or are you instead looking for a curve which causes the light to stay trapped inside indefinitely in time? The latter condition may be harder to specify rigorously when the set of instants at which reflections happen has limit points. $\endgroup$
    – Ricardo Andrade
    Commented Aug 13, 2013 at 21:24
  • 1
    $\begingroup$ The latter condition is just infinite trajectory length... $\endgroup$
    – fedja
    Commented Aug 13, 2013 at 21:31
  • $\begingroup$ @fedja: I guess all that I meant is that it might be hard to define reflection at an instant in time which is a limit point of reflection instants. $\endgroup$
    – Ricardo Andrade
    Commented Aug 13, 2013 at 22:09
  • 1
    $\begingroup$ OK, add "with finitely many reflections on any finite length piece of trajectory" then :). I guess I may have an idea how to capture the rays coming in across $(0.5,1)$ but the whole interval $(0,1)$ is harder. Or, perhaps, I'm talking nonsense. I need some more time to figure out if I have anything interesting to tell :). $\endgroup$
    – fedja
    Commented Aug 13, 2013 at 22:25

1 Answer 1

Reset to default
11
$\begingroup$

I will do it since Fedya has no time.

An example of black hole for a strip of horizontal light rays can be constructed from two arcs of parabolas with common focus and vertical directrixes. black hole with a gap

You can add horizontal mirrors and take a pair of them with reflections in $x$-axis and get the curve you want, say as it shown on the following picture.

black hole without gap

Improve this answer
$\endgroup$
8
  • 1
    $\begingroup$ Cool! I perceived the question as asking about a graph of a smooth function tending to $0$ at $a$ (in which case you should be much more careful and create some structure of focusing and "parallelizing" chambers whose sizes go to $0$ but not too fast as you approach $a$), but, most likely, your example cannot be surpassed in clarity :). $\endgroup$
    – fedja
    Commented Aug 14, 2013 at 2:54
  • $\begingroup$ Very cool. This is very similar to the ellipsoid paradox (see dx.doi.org/10.1119/1.3596430 ), but with one focus taken to infinity. I see now how the physical objections are circumvented. Specifically, with Liouville's theorem, a "packet" of particles gets horizontally spread out as it gets vertically squished and eventually the front of the packet overlaps the back of it so the "phase space" volume taken up by the packet is no longer conserved. $\endgroup$
    – Yoav Kallus
    Commented Aug 14, 2013 at 2:55
  • 3
    $\begingroup$ The volume was 0 to start with: all rays are initially going in one direction ;). $\endgroup$
    – fedja
    Commented Aug 14, 2013 at 2:59
  • $\begingroup$ Yes, :). Still, in this case the rays keep coming back to the space of horizontal rays, so in the measure restricted to that space what I said still makes sense (I think). $\endgroup$
    – Yoav Kallus
    Commented Aug 14, 2013 at 3:03
  • 1
    $\begingroup$ They will but then you just trace their mirror images until they come back to the "physical domain". Or, indeed, just wait until the parabolae intersect by themselves. $\endgroup$
    – fedja
    Commented Aug 14, 2013 at 13:41

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .