Chameleon Bodies

Question

(Note: This was orginally posted on New Year's Eve, 2011.)

Let a body $B$ be a compact set in $\mathbb{R}^3$ with a piecewise smooth boundary. Some pieces/patches of the boundary are perfect mirrors; others perfect matte, colored surfaces. Imagine the view of $B$ from infinity in some direction $u$. A light ray from the "eye" at infinity travels parallel to $u$ until it hits $B$. It then reflects from perfect mirror patches and eventually hits a colored patch, or shoots off (in some direction) to infinity. What is seen in direction $u$ is an array of the colors each ray hits, or transparency when a ray runs to infinity.

More precisely, let $u$ be along the $z$-direction of a Cartesian coordinate system. Each ray parallel to $u$ may be identified by $(x,y)$-coordinates. Each point $(x,y)$ is assigned a color, a positive integer corresponding to the color hit by that ray, or 0 if the ray (ultimately) goes to infinity. The image of $B$ in the $z$-direction is the coloring of $\mathbb{R}^2$. Let $R(u)$ be a region of $\mathbb{R}^2$ large enough to include all the nonzero points of the image from any direction $u$.

As the directions $u$ vary over $\mathbb{S}^2$, the image $R(u)$ changes, generally continuously (say, under the Hausdorff metric). I am interested in bodies $B$ that change discontinuously:

Q1. Does there exist a $B$ whose image changes discontinuously with respect to $u$, preferably with rather dramatic differences, and perhaps several or many such changes?

Such a $B$ could be called a chameleon body, for its appearance changes depending on the viewpoint. Ultimately I would like to control the changes. For example:

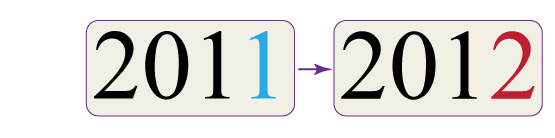
Q2. Is there a $B$ whose view changes between these two images? :-)

---

[This question was inspired by fascinating work by Alexander Plakhov and Vera Roshchina: "Invisibility in billiards" (Nonlinearity 24(3)) and "Bodies invisible from one point" (arXiv:1112.6167). They arrange that a in-ray along line $L$, after ricocheting around inside $B$, emerges and continues along $L$. Orchestrating this for all rays from a point makes $B$ invisible from that point.]

Answer 1

(Too long for a comment.)

Following on from Gerhard's parabolic mirrors suggestion: take a parabolic mirror surface, cut off by a plane perpendicular to the axis of symmetry, so that the resulting surface is still rotationally symmetric. Let's require that the cutoff plane is further out than the focus, so that the focus is not visible from the side of the mirror. Then put a single coloured point at the focus. From exactly one direction, this looks like a solid disk of colour. From any other direction, we either get a single point of colour, or nothing at all. I think that multiple such configurations arranged in a plane, all facing almost the same direction (near perpendicular to the plane) should not interfere with each other (here the focus being hidden from the side is important).

With this set-up, the "2011"/"2012" image could be done with an array of circular "pixels", where half of them show for the 1, and half for the 2. Viewed with less than perfect (ignoring null sets) vision, the single points of the incorrect colour would vanish, and the result would be a (less than) 50% density halftone "newspaper style" image.

I think we can improve on the 50% figure as well. Let's assume that the image we "render" from a given direction is centered on the origin in $\mathbb{R}^3$. We have desired directions for viewing "2011" and "2012", which are very close to each other on $S^2$. No matter how close they are however, we can set "2011" and "2012" back far enough from the origin, in the directions that they are to be viewed in, so that the parabolic mirror pixels for the two images need not be interleaved. Then the density can be made the same as that for circle packing in $\mathbb{R}^2$.

Can it be done with polygonal shapes, so as to get full density of colour? Can it be done so that the images are viewable from subsets of $S^2$ containing open sets?

Answer 2

For question 2, it is not too difficult to construct such an object under your idealized assumptions of perfect mirrors and non-diffracting light.

1. Put a black object that looks like "201" in front of everything, then put a mirror that is shaped like a section of a circular paraboloid in the place of the last digit somewhat further from the observer. This mirror should have focus directly behind one of the black "201" regions, and reflect light from the focus to the observer. The rest of the body will be small, and lie directly behind the black region (thus invisible to the observer).

2. At the focal point, place the edge of a reflective cube. I don't think it is reasonable to define what the observer sees through the mirror at this precise point, since the edge of a cube is a sort of idealization. However, under small perturbations of the observer's position, the observer sees whatever is reflected off one or the other faces of the cube adjacent to the edge. (There are also positions where the observer gathers light from both adjacent faces, due to the failure of the mirror to be a perfect focusing device away from the initial direction, but we may ignore them.)

3. Arrange small objects near the cube so that one of the faces of the cube adjacent to the edge should reflect the figure "1", and the other face should reflect "2".