4
$\begingroup$

Each crossing in a link diagram of a link $L$ has an A-resolution and a B-resolution.

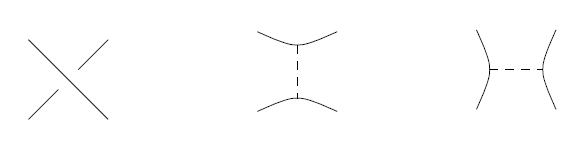
Resolutions of a crossing

Resolving all crossings gives a collection of circles in the plane, connected by dotted lines. A state of a link diagram is a choice for each crossing of either an A-resolution or a B-resolution. The resulting state graph is the graph whose vertices correspond to the circles, and whose edges correspond to dotted lines. A link diagram is said to be semi-adequate if either the state-graph resulting from A-resolving all crossings, or the state-graph resulting from B-resolving all crossings (or both) have no single-edge loops.

In Section 1.3 of Guts of surfaces and the colored Jones polynomial, the authors state that, "The condition that a link be semi-adequate seems to be rather mild". As evidence, they point out several low-crossing-number computations, including a computation of Stoimenow that shows that at least 249,649 of the 253,293 prime knots with 15 crossings are semi-adequate.

The question which lept to my mind (and I have no idea whether this is trivial, well-known, hard, or open) is whether this is an instance of the Strong Law of Small Numbers (which perhaps in this context should be called the `strong law of small links'):

Question: Is a generic link diagram semi-adequate?

When I say generic, I mean in a Kolmogorov complexity sense. Perhaps we choose a random 4-valent (planar) graph, and flip a coin to decide whether each crossing should be over or under.

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Your second paragraph is about knots & links, not diagrams. The answer could be different for a generic knot (chosen randomly from any ordered list of knot types) because a semi-adequate knot generally has many non-semi-adequate diagrams. $\endgroup$
    – Ilya Kofman
    Commented Jul 17, 2013 at 14:24
  • $\begingroup$ Ilya's comment is very much on point. In general, part of what makes this question difficult is that the answer is likely to depend in a huge way on your model of "random knots". Choosing uniformly at random from a list of all $n$-crossing diagrams and choosing uniformly at random from a list of $n$-crossing knots are only two of many different models. Some others: stick knots, knot complements built out of $n$ tetrahedra (Champanerkar-Kofman-Patterson started an enumeration), etc. I don't believe the answer to your question is known for any of these... $\endgroup$
    – Dave Futer
    Commented Jul 17, 2013 at 16:19

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .