Is $ \sum\limits_{n=0}^\infty x^n / \sqrt{n!} $ positive?

Question

Is $$ \sum_{n=0}^\infty {x^n \over \sqrt{n!}} > 0 $$ for all real $x$? (I think it is.) If so, how would one prove this? (To confirm: This is the power series for $e^x$, except with the denominator replaced by $\sqrt{n!}$.)

Answer 1

Looks like the computers really spoiled us :)

GH gave a perfectly valid answer already but the cheapest way to prove positivity is to write $\int_0^1(1-t^n)\log(\frac 1t)^{-3/2}\,\frac{dt}t=c\sqrt n$ with some positive $c$ (just note that the integral converges and the integrand is positive, and make the change of variable $t^n\to t$). Hence $\int_0^1 (f(x)-f(xt))\log(\frac 1t)^{-3/2}\,\frac{dt}t=cxf(x)$. If $x$ is the largest zero of $f$ (which must be negative), then plugging it in, we get $0$ on the right and a negative number on the left, which is a clear contradiction. Thus, crossing the $x$-axis is impossible. Of course, there is nothing sacred about $1/2$. Any power between $0$ and $1$ works just as well.

Answer 2

The affirmative answer follows from my response to this related question.

EDIT. Noam Elkies gave a nicer and more general argument here.

Answer 3

Here is another non-answer. In "Asymptotic Methods in Analysis", chapter 6, de Bruijn proves that $$S(s,n)=\frac{2}{\pi}\Gamma(s)(2ns\log 2n)^{-s}\left(\sin(\pi s)+O\left((\log n)^{-1}\right)\right)$$ where $$S(s,n)= \sum_{k=0}^{2n} (-1)^k \binom{2n}{k}^s$$ for all $0\le s\le\frac{3}{2}$. So at least this explains things asymptotically.

Answer 4

Additional data for Liviu's plots. I used Pari/GP with 1200 digits dec prec, documenting also the required number of terms after which the absolute values of the summands of the series decrease below 1e-100. There seems to be no local minimum...

$\small \begin{array}{rl|r} & & \text{# of terms}\\ x & f(x) & \text{ required} \\ \hline \\ -1 & 0.438599896749 & 201 \\ -2 & 0.247539616819 & 201 \\ -3 & 0.162554775870 & 211 \\ -4 & 0.117399404501 & 257 \\ -5 & 0.0903120618145 & 304 \\ -6 & 0.0726061182760 & 354 \\ -7 & 0.0602796213492 & 407 \\ -8 & 0.0512783927864 & 464 \\ -9 & 0.0444561508357 & 525 \\ -10 & 0.0391295513879 & 589 \\ -11 & 0.0348689168813 & 658 \\ -12 & 0.0313919770798 & 730 \\ -13 & 0.0285063993737 & 808 \\ -14 & 0.0260770215882 & 889 \\ -15 & 0.0240063146159 & 976 \\ -16 & 0.0222222780410 & 1067 \\ -17 & 0.0206706877888 & 1162 \\ -18 & 0.0193099849974 & 1263 \\ -19 & 0.0181078191003 & 1369 \\ -20 & 0.0170386561852 & 1479 \\ -21 & 0.0160820905671 & 1595 \\ -22 & 0.0152216309789 & 1715 \\ -23 & 0.0144438135509 & 1841 \\ -24 & 0.0137375438980 & 1972 \\ -25 & 0.0130936024884 & 2108 \\ -26 & 0.0125042681404 & 2250 \\ -27 & 0.0119630281606 & 2396 \\ -28 & 0.0114643528377 & 2548 \\ -29 & 0.0110035182996 & 2705 \\ -30 & 0.0105764661081 & 2867 \\ -31 & 0.0101796910429 & 3035 \\ -32 & 0.00981015071575 & 3208 \\ -33 & 0.00946519223932 & 3386 \\ -34 & 0.00914249232841 & 3569 \\ -35 & 0.00884000806032 & 3758 \\ -36 & 0.00855593615550 & 3953 \\ -37 & 0.00828867911422 & 4152 \\ -38 & 0.00803681690505 & 4357 \\ -39 & 0.00779908317617 & 4567 \\ -40 & 0.00757434517200 & 4783 \\ -41 & 0.00736158670179 & 5004 \\ -42 & 0.00715989363457 & 5231 \\ -43 & 0.00696844149585 & 5462 \\ -44 & 0.00678648482039 & 5700 \\ -45 & 0.00661334797911 & 5942 \\ -46 & 0.00644841724806 & 6190 \\ -47 & 0.00629113392871 & 6444 \\ -48 & 0.00614098836080 & 6703 \\ -49 & 0.00599751469633 & 6967 \\ -50 & 0.00586028632445 & 7236 \\ -51 & 0.00572891185489 & 7511 \\ -52 & 0.00560303158255 & 7792 \\ -53 & 0.00548231436720 & 8078 \\ -54 & 0.00536645487311 & 8369 \\ -55 & 0.00525517112099 & 8666 \\ -56 & 0.00514820231209 & 8968 \\ -57 & 0.00504530688991 & 9275 \\ -58 & 0.00494626080983 & 9588 \\ -59 & 0.00485085599129 & 9907 \\ -60 & 0.00475889893049 & 10230 \\ -61 & 0.00467020945455 & 10560 \\ -62 & 0.00458461960073 & 10894 \\ -63 & 0.00450197260623 & 11234 \\ -64 & 0.00442212199624 & 11580 \\ -65 & 0.00434493075923 & 11931 \\ -66 & 0.00427027059992 & 12287 \\ -67 & 0.00419802126157 & 12649 \\ -68 & 0.00412806991028 & 13016 \\ -69 & 0.00406031057475 & 13388 \\ -70 & 0.00399464363573 & 13766 \end{array} $

Answer 5

This comment serves to record a partial attempt, which didn't get very far but might be useful to others. Following a suggestion of Mark Wildon and Arthur B, define $$f_n(\alpha) := \sum (-1)^r \binom{n}{r}^{\alpha}.$$ This is zero for $n$ odd, so we will assume $n$ is even from now on.

Mark Wildon shows that it would be enough to show that $f_n(1/2) \geq 0$ for all $n$. It is easy to see that $f_n(0) = 1$ and $f_n(1)=0$. Arthur B notes that, experimentally, $f_n(\alpha)$ appears to be decreasing on the interval $[0,1]$. If we could prove that $f_n$ was decreasing, that would of course show that $f_n(1/2) > f_n(1) =0$.

I had the idea to break this problem into two parts, each of which appears supported by numerical data:
1. Show that $f_n$ is convex on $[0,1]$.
2. Show that $f'_n(1) < 0$.
If we establish both of these, then clearly $f_n$ is decreasing.

I have made no progress on part 1, but here is most of a proof for part 2. We have $$f'_n(1) = \sum (-1)^r \binom{n}{r} \log \binom{n}{r} = \sum (-1)^r \binom{n}{r} \left( \log(n!) - \log r!- \log (n-r)! \right)$$ $$=-2 \sum (-1)^r \binom{n}{r} \left( \log(1) + \log(2) + \cdots + \log (r) \right)$$ $$=-2 \sum (-1)^r \binom{n-1}{r} \log r.$$ At the first line break, we combined the $r!$ and the $(n-r)!$ terms (using that $n$ is even); at the second, we took partial differences once.

This last sum is evaluated asymptotically in this math.SE thread. The leading term is $\log \log n$, so the sum is positive for $n$ large, and $f'_n$ is negative, as desired. The sole gap in this argument is that the math.SE thread doesn't give explicit bounds, so this proof might only be right for large enough $n$.

This answer becomes much more interesting if someone can crack that convexity claim.

Answer 6

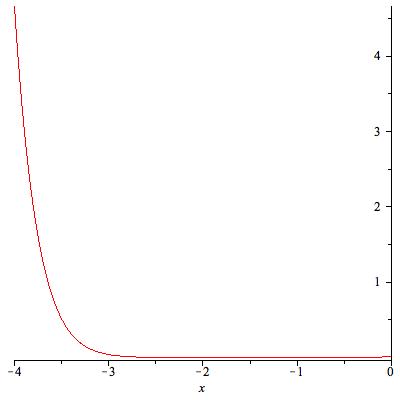
Here is a plot of

$$\frac{1}{100}\left(\sum_{k=0}^{16}\frac{x^k}{\sqrt{k!}}\right)$$

on the interval $[-4,0]$. (Above I added the terms up to degree $16$.)

Next, is a plot of

$$\frac{1}{100}\left(\sum_{k=0}^{15}\frac{x^k}{\sqrt{k!}}\right)$$

on the interval $[-3,0]$. (Above I added the terms up to degree $15$)

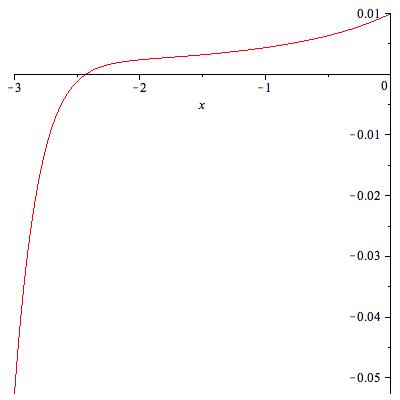
This is one strange series.

Answer 7

Another "not-yet-answer"...

I've tried another idea. Assume the function f(x) is expressed by the following composition: $$\small x' = \exp(x)-1 $$ $$\small f(x) = g(x') = g(exp(x)-1) $$ The idea is, that the unavoidable big "hump" in the partial sums, after which the sequence of partial sums begins to decrease, may be absorbed by the function $\small g(x)$ - because $\small \exp(x) $ is really small for large negative x and x' is then very little above -1. I did not yet arrive at a conclusive result; but the power series for $\small g(x) $ begins with the smooth looking form (and gives the partial sums for $\small x'=\exp(-100)-1 $):
$\qquad \small \begin{array} {r|r} \text{powerseries} & \text{partial sums for x' } \\ \hline \\ 1.00000000000 & 1.00000000000 \\ +1.00000000000x & 3.72007597602E-44 \\ +0.207106781187x^{2} & 0.207106781187 \\ +0.0344748426106x^{3} & 0.172631938576 \\ -0.0100670743762x^{4} & 0.162564864200 \\ +0.00821765977664x^{5} & 0.154347204423 \\ -0.00654357122833x^{6} & 0.147803633195 \\ +0.00537330847179x^{7} & 0.142430324723 \\ -0.00451702185603x^{8} & 0.137913302867 \\ +0.00386915976824x^{9} & 0.134044143099 \\ -0.00336528035075x^{10} & 0.130678862748 \\ +0.00296428202807x^{11} & 0.127714580720 \\ -0.00263893325448x^{12} & 0.125075647465 \\ +0.00237058888853x^{13} & 0.122705058577 \\ -0.00214611388717x^{14} & 0.120558944690 \\ +0.00195602261228x^{15} & 0.118602922077 \\ -0.00179331457091x^{16} & 0.116809607506 \\ +0.00165272361723x^{17} & 0.115156883889 \\ -0.00153022060566x^{18} & 0.113626663284 \\ +0.00142267593977x^{19} & 0.112203987344 \\ -0.00132762563657x^{20} & 0.110876361707 \\ +0.00124310598493x^{21} & 0.109633255722 \\ -0.00116753462507x^{22} & 0.108465721097 \\ +0.00109962364925x^{23} & 0.107366097448 \\ \end{array} $

The the question is, for some large negative x, say $\small x=-100 \qquad x'=exp(-100)-1 = -1+ \epsilon $ the series $\ g(x') $ converges to zero. Unfortunately - although we've translated the original problem to one with nice small numbers I don't see, how to really come nearer a solution, because the convergence of $\small g(-1+\epsilon) $ is really slow - if it converges at all to a positive value... So this is not yet a solution, but perhaps a suggestion for a path to try...

Answer 8

Although the following does not provide another proof (perhaps it is possible to attempt one on this basis) I found it nice to see the following pictures.
Let's take from the series $f(x) = \sum_{k=0}^\infty {x^k \over \sqrt{k!}}$ the following variants in the same spirit as we have the hyperbolic and trigonometric series from the exponential-series: $$\begin{array}{} \small \exp_{\tiny \sqrt{\,}}(x) &=& f(x) \\ \small \cosh_{\tiny \sqrt{\,}}(x) &=& \sum_{k=0}^\infty {x^{2k} \over \sqrt{(2k)!}} \\ \small \sinh_{\tiny \sqrt{\,}}(x) &=& \sum_{k=0}^\infty {x^{2k+1} \over \sqrt{(2k+1)!}} \\ \small \tanh_{\tiny \sqrt{\,}}(x) &=& { \sinh_{\tiny \sqrt{\,}}(x)\over \cosh_{\tiny \sqrt{\,}}(x) } \\ \small \cos_{\tiny \sqrt{\,}}(x) &=& \sum_{k=0}^\infty (-1)^k {x^{2k} \over \sqrt{(2k)!}} \\ \small \sin_{\tiny \sqrt{\,}}(x) &=& \sum_{k=0}^\infty (-1)^k {x^{2k+1} \over \sqrt{(2k+1)!}} \\ \end{array}$$ The answer to your question is equivalent to say, that always (="for real $x$")

• $\small \cosh_{\tiny \sqrt{\,}}(x)$ is larger than $\small \sinh_{\tiny \sqrt{\,}}(x) $ $\qquad \qquad$ or that
• $\small \mid \tanh_{\tiny \sqrt{\,}}(x) \mid \lt 1$

---

To illustrate this I've plotted the $\sinh_{\tiny \sqrt{\,}}$ and $\cosh_{\tiny \sqrt{\,}}$-curves:

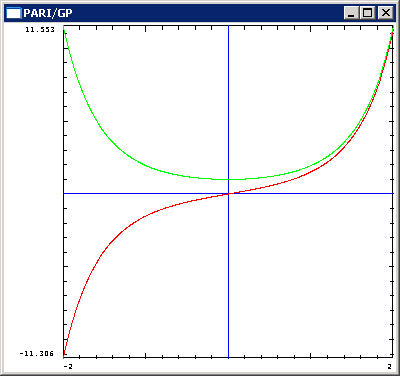
This gives surely an extremely familiar impression...

The $\tanh_{\tiny \sqrt{\,}}$-curve looks completely familiar too:

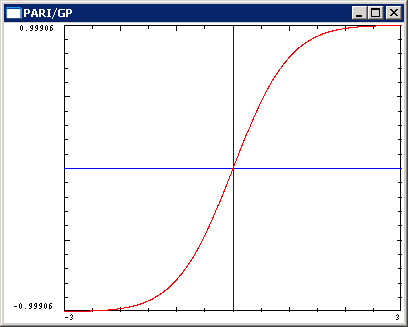
and the image suggests, that indeed the absolute value of $\small \tanh_{\tiny \sqrt{\,}}(x) $ very likely is smaller than $1$ for all real $x$.

---

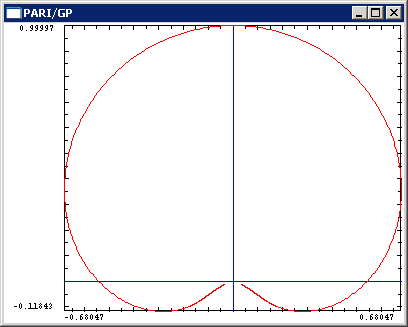
However, things are different for the $\sin_{\tiny \sqrt{\,}}$ and $\cos_{\tiny \sqrt{\,}}$ curves - they deviate strongly from the nicely periodic common trigonometric functions:

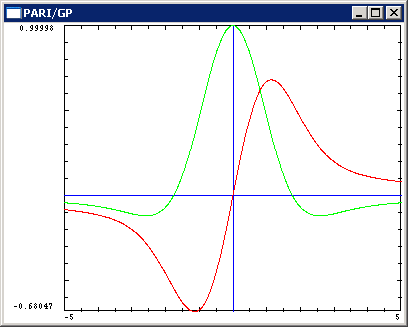
and combined they do not give a circle, but some ugly thing, strongly distorted (y-axis by $\small \cos_{\tiny \sqrt{ \,} }(\phi)$, x-axis by $\small \sin_{\tiny \sqrt{ \,} }(\phi)$, $\phi$ from $-5$ to $+5$) :

---