I have a probability distribution defined by the following density function:
$f(k,j,n,m)=\frac{(m n)! \mathcal{S}_k^{(j)}}{(m n)^k (m n-j)!}$ (With $\mathcal{S}_k^{(j)}$ being the Stirling number of the second kind.)
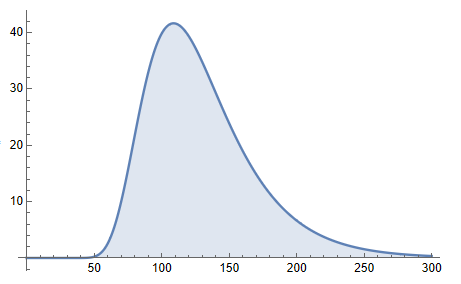
Here you can see a sample plot for $j=29,n=30,m=1$, with $k$ being the horizontal axis:
My goal is to calculate its mean to get an expected value for $k$, but when I apply the definition I get the following expression:
$\sum _{k=1}^{\infty } \frac{k (m n)! \mathcal{S}_k^{(j)} (m n)^{-k}}{(m n-j)!}$
How can I solve this summation so that it can provide a resulting expression as a function of $j,n$ and $m$?