In one line: Given an exponentially decaying sine wave $x(t)$, how can we predict the amplitude of the resulting peak in frequency spectrum using discrete Fourier transform.
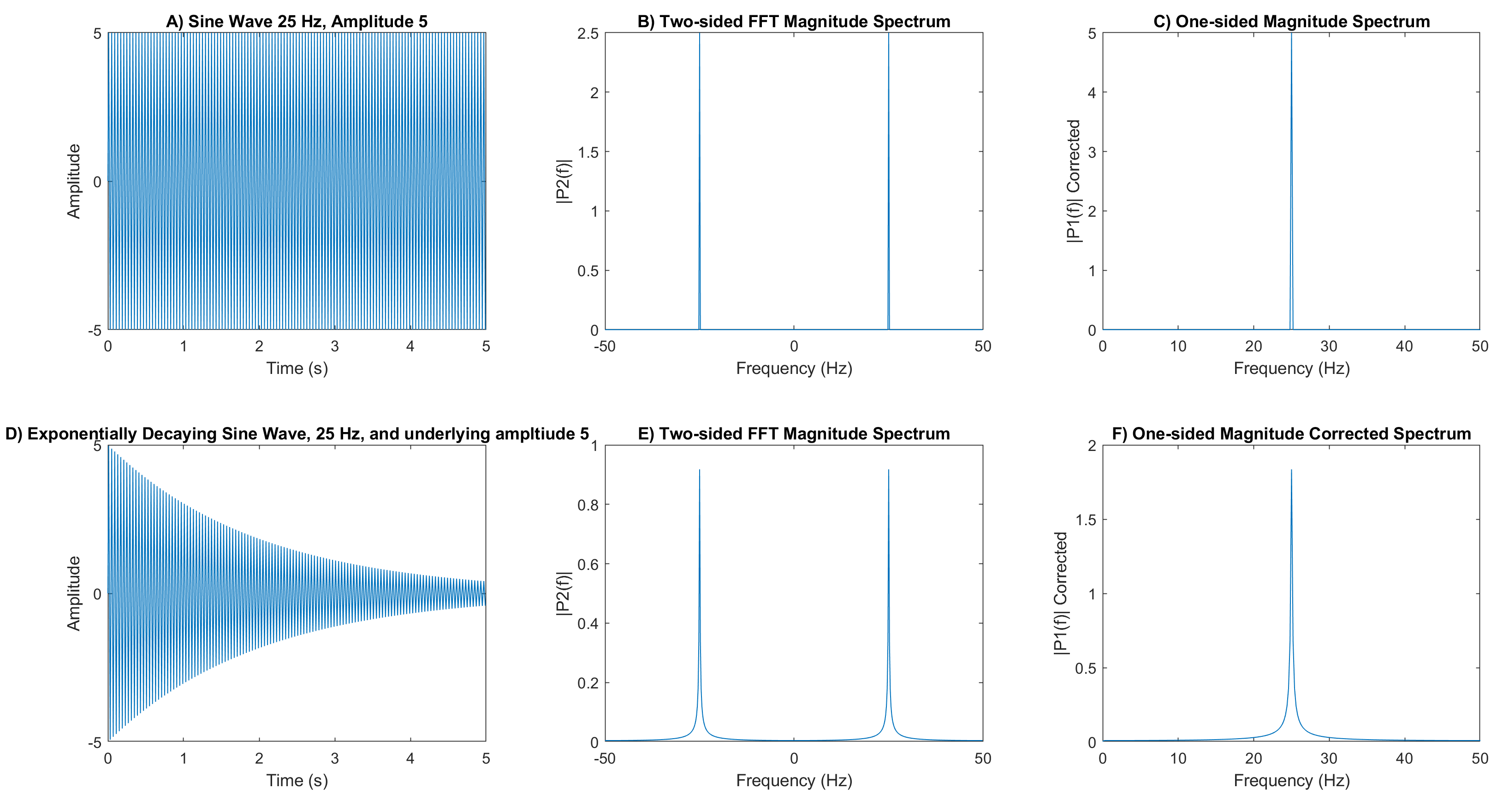
In nuclear magnetic resonance experiments, the raw data is an exponentially decaying sinuoid(s) as a function of time (t $\geq$ 0). In order to see the frequency spectrum the standard protocol is to do a Fast Fourier Transform (FFT) and display one-sided frequency spectrum 1.
a) Simple scenario: We have a non-decaying sine wave of frequency $\omega_0$ and amplitude $A$. FFT of this signal is two peaks (one corresponding to positive frequency and the other one at negative frequency situated at $\omega_0$ and amplitude $A/2$. Note that we have to normalize the FFT with the number of sampled points in order to get the right amplitudes in the frequency spectrum.
b) Real experimental scernaio: Let us say we have an exponentially decaying sine wave $x(t)$, then,
$$x(t) = A \sin(\omega_0 t) e^{-\alpha t} \, u(t)$$
where $u(t)$ is the Heaviside unit step function, $\alpha$ is positive and real. Now the key question is that in (a), we were able to predict the amplitude of the peaks in the frequency spectrum. It was $A/2$. In this exponentially decaying case, is there a way to predict the amplitude of the resulting peak in frequency spectrum?
An annotated figure will clarify the question. Starting from (a) a sine wave with 25 Hz and amplitude of 5 will show up as a single peak in FFT spectrum of amplitude 5/2 (two sided version) or 5 in the one-sided spectrum.
Now the same sinusoid is multiplied by an exponential with $\alpha$= 0.5. We get a much less peak height in the frequency spectrum. In short, is there a way to predict this peak height if we know the decay constant and initial amplitude of the sine wave in FFT. Thanks.

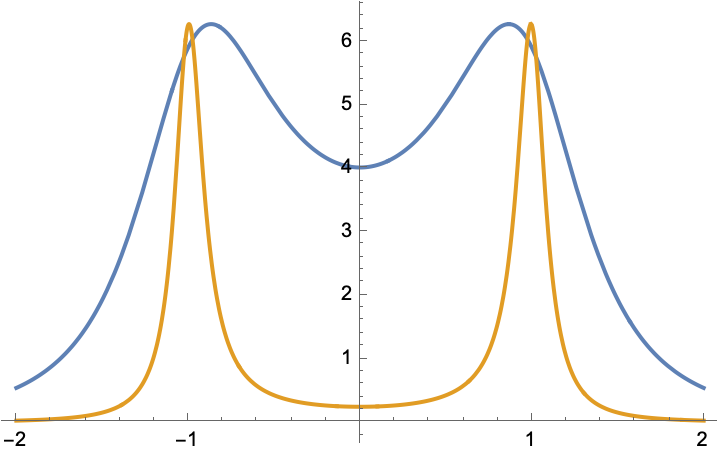
fftcommand for the Fourier transform where the exponentially dampened signal is defined asA*exp(-alpha* t) .*sin(2 * pi * frequency * t). Regardless of the size of thealphavalue, there is no shift in the frequency domain at all. $\endgroup$