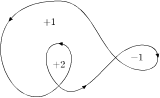
As the title suggests, I am trying to find something like an isoperimetric inequality for smooth immersions of the 2-sphere into $\mathbb{R}^3$ that relates the surface area to the enclosed 3d-volume. There are a couple of issues here: firstly, does it make sense to talk about an 'inside' and 'outside' of a possibly self-intersecting surface in $\mathbb{R}^3$? My solution to this was to just consider the algebraic volume [1,2]: $$ V=\frac{1}{3}\int_M\langle N, x\rangle dM, $$ where $x:M\rightarrow\mathbb{R}^3$ is the immersion and $N$ is the orientation. I would like to show some kind of inequality relating $V$ to the surface area of the immersion, $$ A=\int_M d\Sigma $$ where $d\Sigma$ is the induced area element. My idea so far has been to try and modify Gromov's proof of the isoperimetric inequality using Stokes' theorem [3], but I haven't made much progress. Any helpful references would be appreciated.
EDIT:
In [4] and [5] the following isoperimetric inequality is presented:
$$
\left(\sum_{k} n_k \mathscr L(W_k)\right)^2\leq \left(\sum_{k} |n_k| \mathscr L(W_k)\right)^2 \leq (36\pi)^{-1}A^3
$$
where $W_k$ denote the various connected components of $\mathbb{R}\setminus x(M)$, and $n_k$ are the (generalised) winding numbers of $W_k$. Here, $\mathscr L(W_k)$ denotes the 3d-Lebesgue volume of $W_k$.
References:
[1] López, Rafael. Constant mean curvature surfaces with boundary. Springer Science & Business Media, 2013. (Chapter 2)
[2] Andrews, Ben, et al. Extrinsic Geometric Flows. American Mathematical Society, 2020. (Chapter 5)
[3] Berger, Marcel. Geometry II. Springer Science & Business Media, 2009. (12.11.4)
[4] Osserman, Robert. "The isoperimetric inequality." Bulletin of the American Mathematical Society 84.6 (1978): 1182-1238.
[5] Rado, Tibor. "The isoperimetric inequality and the Lebesgue definition of surface area." Transactions of the American Mathematical Society 61.3 (1947): 530-555.