1
$\begingroup$

References:

  1. https://math.stackexchange.com/questions/1838617/dividing-an-equilateral-triangle-into-n-equal-possibly-non-connected-parts

  2. On congruent partitions of planar regions

  3. https://research.ibm.com/haifa/ponderthis/challenges/December2003.html

Question 1: Given a number $N$, can we construct a convex planar region that can be cut into $N$ mutually congruent, connected, convex pieces but not into any other number of connected, mutually congruent convex pieces?

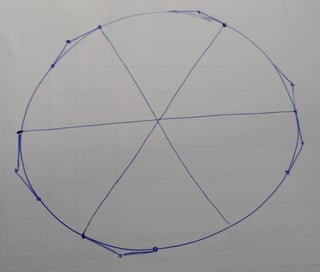
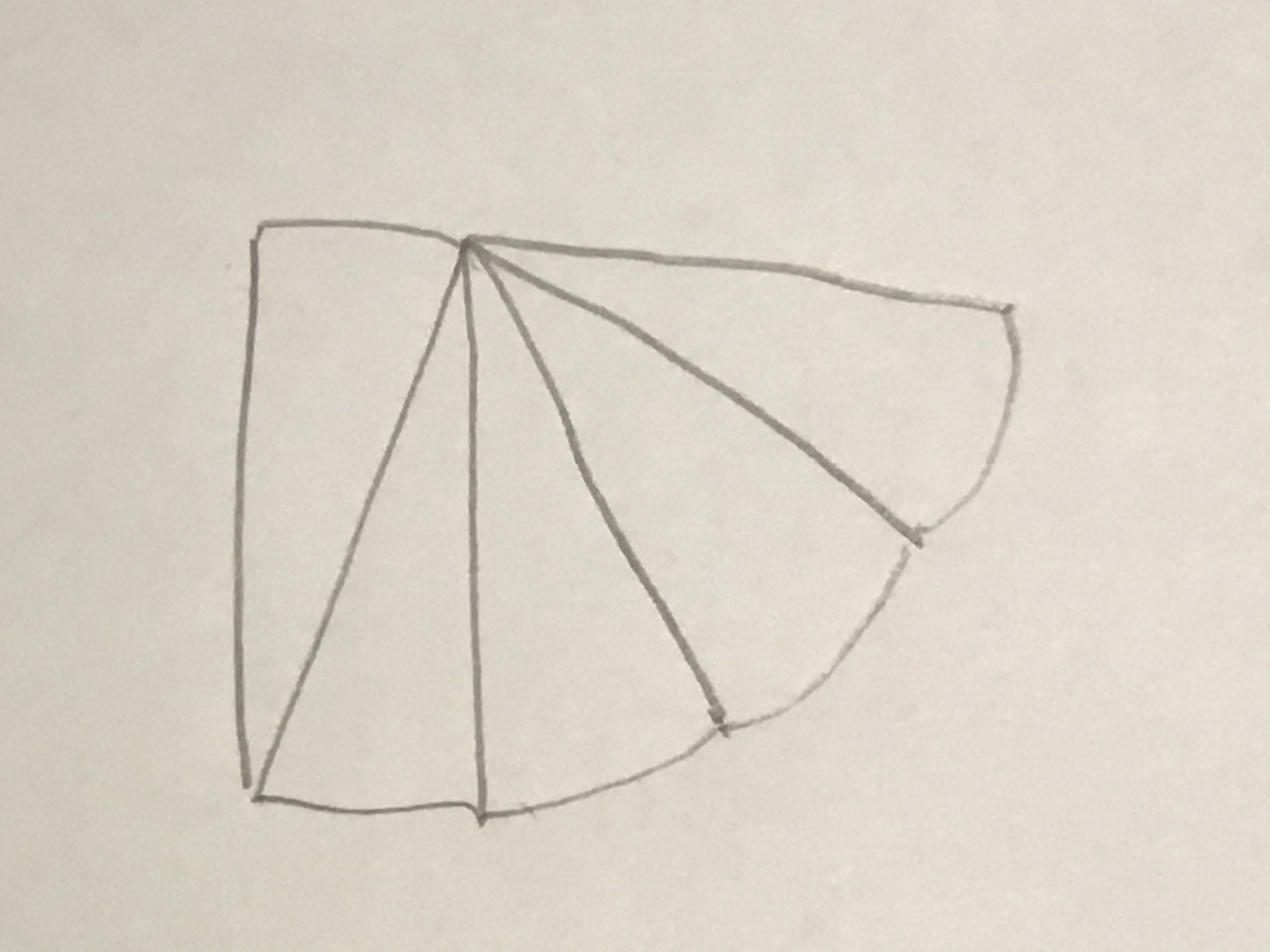
Partial Answer (guess): For prime $N$, there seems to be a simple way. Take a regular $N$-gon and mark from it $N$ mutually congruent quadrilaterals by drawing lines from center to mid points of the N faces. Now in each quadrilateral, replace the two 'outward' edges by copies of a polyline with say $p$ edges and with angles that are irrational fractions of $\pi$ (see ref 3 for some justification for 'irrational') in such a way that the $N$-gon becomes a convex $Np$-gon. This $Np$-gon seems to allow partition into $N$ and only $N$ pieces that are mutually congruent, convex and connected.

Remark: As per the answers below, one can upgrade above attempt to work for all values of $N$, not only primes.

Question 2: Are there convex planar regions that allow partition into mutually congruent and connected pieces only when the number of pieces is one of exactly $2$ specified values — for example, is there a convex region that can only be cut into $3$ connected congruent pieces or $5$ congruent pieces but not into any other number of congruent pieces?

Remark: Answer to question 1 can be slightly modified to yield planar regions that seem to allow partition into only $N$ mutually congruent pieces or $kN$ mutually congruent pieces where $N$ and $k$ are primes.

Note: One can widen question 2 and ask if given a set S of numbers relatively prime to one another, one can construct a planar region that allows partition only into sets of congruent pieces with cardinalities equal to each element in set S and no other number. One can also consider less constrained versions - eg. allow the mutually congruent pieces and the input region to be non-convex.

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ I think it's inappropriate to widen a question after two answers have already been given -- instead, it would be appropriate to roll back to v4 of the question, accept one of the answers, and then possibly ask another question on the same theme. $\endgroup$
    – user44143
    Commented Jan 13, 2022 at 16:54
  • 1
    $\begingroup$ Please note that you can accept an answer by clicking on the check mark below the vote score (accepting satisfactory answers is part of the site etiquette). $\endgroup$
    – Stefan Kohl ♦
    Commented Jan 13, 2022 at 18:14
  • $\begingroup$ thanks stefan kohl. and thanks matt f. i just accepted the answers - i didn't know how to do it earlier - and i shall go back and accept some earlier answers! reg. the other point, question 2, which remains, was there from the beginning of the post and i didn't really widen the question. $\endgroup$
    – Nandakumar R
    Commented Jan 14, 2022 at 12:15

2 Answers 2

Reset to default
2
$\begingroup$

This construction (the picture has the case $N=6$) seems to work. It is obtained in 2 steps:

-Begin with a convex set formed by $N$ equal pieces, each having a boundary formed by two segments and a piece of circumference.

-Change the orientation of one piece.

enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ I agree. This widens the answer for question 1 from prime N - assuming that mirror images are congruent. If we treat mirror images as non-congruent, what could one say? Shall add a remark in the question. Thanks. $\endgroup$
    – Nandakumar R
    Commented Jan 12, 2022 at 9:47
3
$\begingroup$

What if you take $N$ thin slices of pizza (as in your example, but thinner) and arrange them like this?enter image description here

Improve this answer
$\endgroup$
3
  • $\begingroup$ This could be divided into $kN$ pieces for any $k$. But if you change the slices by strange slices like the ones from my answer it seems to work without orientation changes $\endgroup$
    – Saúl RM
    Commented Jan 12, 2022 at 10:05
  • 1
    $\begingroup$ @SaúlRodríguezMartín That’s what I meant by ‘as in your example’ —- meaning the OP’s example, with a polyline of $p$ segments. You know, a pizza is not perfectly round;) $\endgroup$
    – Ilya Bogdanov
    Commented Jan 12, 2022 at 10:07
  • $\begingroup$ Guess this rearrangement with one piece going the other way - but with all pieces being congruent - could work for any N, including all composites. IOW, this polygon appears to be partitionable only into N congruent pieces. And it seems to have no problem with mirrors. thnx! $\endgroup$
    – Nandakumar R
    Commented Jan 12, 2022 at 10:57

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .