The question is based on the proof of the main theorem of Gabai's paper on Foliations and the topology of 3-manifolds which is the following:
Theorem 5.1. Suppose $M$ is connected, and $(M,\gamma)$ has a sutured manifold hierarchy $$(M,\gamma) = (M_0,\gamma_0)\xrightarrow{S_1}(M_1,\gamma_1)\to\cdots\xrightarrow{S_n}(M_n,\gamma_n)$$ so that no component of $R(\gamma_i)$ is a compressing torus. Then there exist transversely oriented foliations $\mathcal{F}_0$ and $\mathcal{F}_1$ of $M$ such that the following hold.
$(1)$ $\mathcal{F}_0$ and $\mathcal{F}_1$ are tangent to $R(\gamma)$.
$(2)$ $\mathcal{F}_0$ and $\mathcal{F}_1$ are transverse to $\gamma$.
$(3)$ If $H_2(M,\gamma)\neq\emptyset$, then every leaf of $\mathcal{F}_0$ and $\mathcal{F}_1$ nontrivially intersects a transverse closed curve or a transverse arc with endpoints in $R(\gamma)$. However, if $\emptyset\neq\partial M = R_+(\gamma)$ or $R_-(\gamma)$, then this holds only for interior leaves.
$(4)$ There are no 2-dimensional Reeb components on $\mathcal{F}_i|\gamma,i=0,1$.
$(5)$ $\mathcal{F}_1$ is $C^\infty$ except possibly along Toral components of $R(\gamma)$ or $S_1$ if $\partial M = \emptyset$.
$(6)$ $\mathcal{F}_0$ is of finite depth.
The question is during the construction of $\mathcal{F}_1^{i-1}$ in case 2, i.e., $\partial T_i$ is contained in a component $V$ of $R(\gamma_i)$.
I'll follow the proof written in the paper: To construct $\mathcal{F}_1^{i-1}$ in this case, first glue $T_i^+$ and $T_i^-$ and produce a manifold $Q$ with an induced foliation $\mathcal{F}^1$ via $\mathcal{F}_1^i$. Let $f$ be the holonomy of the transverse annulus.
For $f = 1$ then following the construction of $\mathcal{F}_0^{i-1}$ get $C^\infty$ foliation. For $f\neq 1$ case, one can "push the holonomy to the boundary" to reduce the case to $f = 1$ case. The main part I'm not convinced is case 4:
If $V$ is a closed oriented surface of genus $>1$, and $f\neq 1$ then we need the following theorem.
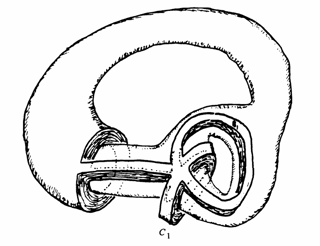
Theorem (Mather-Sergeraert-Thurston). If $f:I\to I$ is a $C^\infty$ diffeomorphism satisfying $${d^n f\over dt^n}(\alpha) = \begin{cases} 1, & n=1,\\ 0, & n>1,\end{cases}$$ for $\alpha\in\{0,1\}$, then there exists $C^\infty$ diffeomorphisms $c_i,b_i:I\to I$, $i =1,2,\ldots,n$, satisfying the above conditions so that $$f\circ[c_1,b_1]\circ\cdots[c_n,b_n] = 1.$$ $\quad$Now let $Q_1$ be obtained by attaching thick bands $B_1$ and $C_1$ to $\partial Q$. Extend $\mathcal{F}^1$ to $\mathcal{F}^2$ on $Q_1$ by foliating these bands so that the holonomy along $B_1$ (or $C_1$) is $b_1$ (or $c_1$). One now observes that the holonomy along the new transverse annulus is $fc_1b_1c_1^{-1}b_1^{-1} = f\circ [c_1,b_1]$. By repeating this procedure $n$ times one gets a foliation $\mathcal{F}^{n+1}$ on $Q_n$ whose holonomy along the transverse annulus is trivial.
Question(s)
(1) In the last sentence, it says that repeating this procedure $n$ times. I understand the $n=1$ case by the drawing in the paper but I'm not convinced how the repetition can be done. How about the $n=2$ case or higher?
(2) It's a relatively minor question but I think the assumption of theorem 5.1 that *no component of $R(\gamma_i)$ is a compressing torusno component of $R(\gamma_i)$ is a compressing torus is just to avoid possible Reeb foliation? I don't understand why that assumption is required. It seems it's not specifically mentioned in the proof.