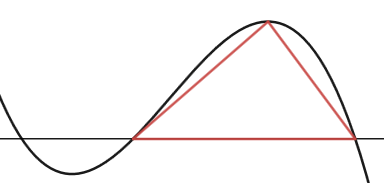
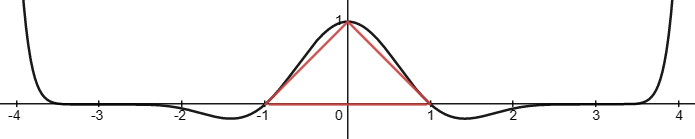
A triangle is drawn in a region bounded by the $x$-axis and a polynomial curve with all real roots, with one side of the triangle on the $x$-axis, as shown in this example:
What is the supremum of the ratio $R$ of the triangle's area to the region's area?
(We require that the polynomial has all real roots, because otherwise the answer would be trivial: the supremum would be $1$, for example with $y=x-x^n$ and letting $n\to\infty$.)
I considered the largest area triangle drawn in the region bounded by the $x$-axis and the polynomial $y=(-1)^{n-1}(x^2-1)\left(\left(\frac{x}{a}\right)^2-1\right)^n, a>1$, from $x=-1$ to $x=1$.
Numerical investigation suggests that for any given $a$ value, the ratio $R$ is maximized when $n\approx 1.88a^2$$n\approx 1.880001a^2$.
$\color{blue}{a=10}$:
$\space R\approx0.8953810$ with $y=(x^2-1)\left(\left(\frac{x}{10}\right)^2-1\right)^{\color{red}{187}}$
$\space R\approx0.8953823$ with $y=-(x^2-1)\left(\left(\frac{x}{10}\right)^2-1\right)^{\color{red}{188}}$
$\space R\approx0.8953822$ with $y=(x^2-1)\left(\left(\frac{x}{10}\right)^2-1\right)^{\color{red}{189}}$
$\color{blue}{a=100}$:
$\space R\approx0.8955295$ with $y=-(x^2-1)\left(\left(\frac{x}{100}\right)^2-1\right)^{\color{red}{18799}}$
$\space R\approx0.8955296$ with $y=(x^2-1)\left(\left(\frac{x}{100}\right)^2-1\right)^{\color{red}{18800}}$
$\space R\approx0.8955295$ with $y=-(x^2-1)\left(\left(\frac{x}{100}\right)^2-1\right)^{\color{red}{18801}}$
$\color{blue}{a=1000}$:
$\space R\approx0.8955309$$\space R\approx0.89553095$ with $y=(x^2-1)\left(\left(\frac{x}{1000}\right)^2-1\right)^{\color{red}{1879990}}$$y=(x^2-1)\left(\left(\frac{x}{1000}\right)^2-1\right)^{\color{red}{1880000}}$
$\space R\approx0.8955311$$\space R\approx0.89553105$ with $y=(x^2-1)\left(\left(\frac{x}{1000}\right)^2-1\right)^{\color{red}{1880000}}$$y=(x^2-1)\left(\left(\frac{x}{1000}\right)^2-1\right)^{\color{red}{1880001}}$
$\space R\approx0.8955310$$\space R\approx0.89553101$ with $y=(x^2-1)\left(\left(\frac{x}{1000}\right)^2-1\right)^{\color{red}{1880010}}$$y=(x^2-1)\left(\left(\frac{x}{1000}\right)^2-1\right)^{\color{red}{1880002}}$
I conjecture that the supremum of $R$$\text{sup}(R)$ is approached when $a\to\infty$ (for each value of $a$, we choose the value of $n$ that maximizes $R$), and that $R\approx 0.8955$$\text{sup}(R)\approx 0.895531$.