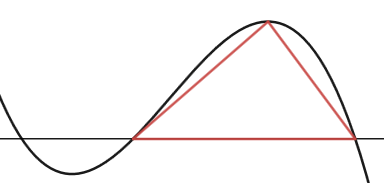
A triangle is drawn in a region bounded by the $x$-axis and a polynomial curve with all real roots, with one side of the triangle on the $x$-axis, as shown in this example:

What is the supremum of the ratio of the triangle's area to the region's area?

(We require that the polynomial has all real roots, because otherwise the answer would be trivial: the supremum would be $1$, for example with $y=x-x^n$ and letting $n\to\infty$.)

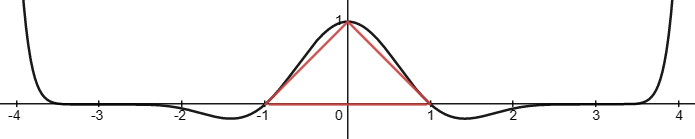
I hypothesize that the supremum of the ratio is approached with a region bounded by the $x$-axis from $x=-1$ to $x=1$, and a polynomial of the form $y=(\pm)(x^2-1)(x^2-r^2)^n, r>1$, where for any given $r$ value, the ratio is maximized with a certain $n$ value.

For example, when $r=5$, the ratio $R$ is maximized when $n=47$:

$R\approx0.894915$ with $y=-(x^2-1)(x^2-5^2)^{\color{red}{46}}$

$R\approx0.894934$ with $y=(x^2-1)(x^2-5^2)^{\color{red}{47}}$

$R\approx0.894933$ with $y=-(x^2-1)(x^2-5^2)^{\color{red}{48}}$