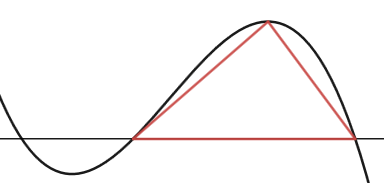
A triangle is drawn in a region bounded by the $x$-axis and a polynomial curve with all real roots, with one side of the triangle on the $x$-axis, as shown in this example:

What is the supremum of the ratio of the triangle's area to the region's area?

(We require that the polynomial has all real roots, because otherwise the answer would be trivial: the supremum would be $1$, for example with $y=x-x^n$ and letting $n\to\infty$.)

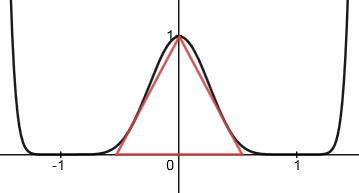
I hypothesize that the supremum of the ratio is approached with a region bounded by the $x$-axis from $x=-1$ to $x=1$, and $y=(\pm)(x^2-1) \prod\limits_{k=1}^n \left(\left(\frac{x}{r_k}\right)^2-1\right), r_k\ge1$, $r_k$'s not necessarily unique.

Using this hypothesis, the largest ratio $R$ that I have found is

$R\approx0.888005$ with $y=(x^2-1)^6 \left(\left(\frac{x}{10}\right)^2-1\right)^{50} \left(\left(\frac{x}{100}\right)^2-1\right)^{50}$

(I'm using desmos, which does not handle very large exponents.)

Could the supremum of the ratio be $8/9$ ?

Strangely, if we adjust the polynomial above, by increasing the power of the $(x^2-1)$ factor, the ratio decreases after the power reaches $6$.

$R\approx0.887937$ with $y=(x^2-1)^{\color{red}{5}} \left(\left(\frac{x}{10}\right)^2-1\right)^{50} \left(\left(\frac{x}{100}\right)^2-1\right)^{50}$

$R\approx0.888005$ with $y=(x^2-1)^{\color{red}{6}} \left(\left(\frac{x}{10}\right)^2-1\right)^{50} \left(\left(\frac{x}{100}\right)^2-1\right)^{50}$

$R\approx0.887917$ with $y=(x^2-1)^{\color{red}{7}} \left(\left(\frac{x}{10}\right)^2-1\right)^{50} \left(\left(\frac{x}{100}\right)^2-1\right)^{50}$

$R\approx0.885052$ with $y=(x^2-1)^{\color{red}{50}} \left(\left(\frac{x}{10}\right)^2-1\right)^{50} \left(\left(\frac{x}{100}\right)^2-1\right)^{50}$

(I posted this question on Math SE, but it received no answer, and few views. I hope that it may be of interest to some people here.)

EDIT

I found a larger ratio:

$R\approx0.894875$ with $y=-(x^2-1) \left(\left(\frac{x}{5}\right)^2-1\right)^{50} \left(\left(\frac{x}{100}\right)^2-1\right)^{50}$