On MSE I asked whether each of $\int_0^\infty\frac{dx}{x^x},\int_0^\infty\frac{dx}{x^{x^{x^x}}},\int_0^\infty\frac{dx}{x^{x^{x^{x^{x^x}}}}},\cdots$ was less than $2$ and received answers on bounding the tetration integrals by a smaller-order power tower, but the numerical results were attained computationally.
While I don't know whether a simple, analytical proof exists to prove the upper bound of $2$, I was especially curious as to why the integrals from $1/x^{x^{x^x}}$ onwards seemed to form a monotonically increasing sequence

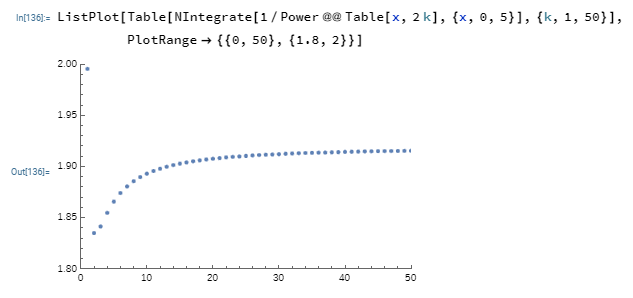
Is this observation true? That is, if $f_0(x)=x^x$ and $f_{k+1}(x)=x^{x^{f_k(x)}}$, then $$\int_0^\infty{dx\over f_k(x)}<\int_0^\infty{dx\over f_{k+1}(x)}$$ for all $k\ge1$ which can be interpreted as the area gained over $(0,1)$ is greater than that lost over $(1,\infty)$.
Wolfram Cloud gives these consecutive differences. It appears that these differences decay on the order of $x^{-\log x}$, and decrease monotonically in most instances as well.