This is a problem from page 3 of Bourgain, Burq, and Zworski - Control for Schrödinger equations on 2-tori: rough potentials, the author claim that
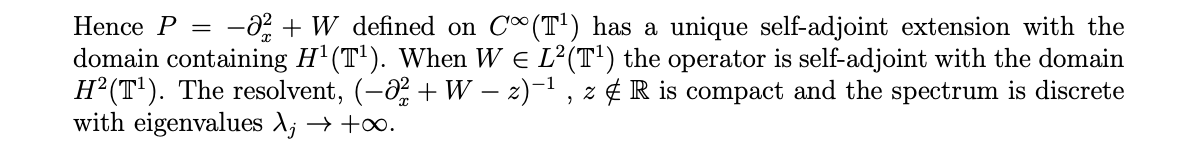
Hence $P = - \partial_x^2 + W$ defined on $C^\infty(\mathbb{T}^1)$ has a unique self-adjoint extension with the domain containing $H^1(\mathbb{T^1})$. When $W \in L^2(\mathbb{T}^1)$ the operator is self-adjoint with the domain $H^2(\mathbb{T^1})$. The resolvent, $(-\partial_x^2+W-z)^{-1}$, $z \notin \mathbb{R}$ is compact and the spectrum is discrete with eigenvalues $\lambda_j \to + \infty$.
Problem. Let the differential operator $P=-\partial_x^2+W(x)$ be defined on $D(P)=H^2(S^1)\subset L^2(S^1)$, if $W\in L^2(S^1)$, then $P$ is self-adjoint.
By definition, $v\in D(P^*)$ if and only if there exists $C(v)>0$ such that $\lvert\langle Pu,v\rangle_{L^2}\rvert\leq C(v)\lVert u\rVert_{L^2}$ for all $u\in D(P)$. On the one hand, it is easy to show that $H^2(S^1)\subset D(P^*)$ by integration by parts, on the other hand, if $v\in D(P^*)$, then there exists $P^*v\in L^2(S^1)$ such that for $u\in D(P)$ $$\langle P^*v,u\rangle=\langle v,Pu\rangle =\int_{S^1} v(x)(-\partial^2_xu(x)+W(x)u(x))\,dx,$$ in the sense of distribution, we get $P^*v=-\partial_x^2 v+W(x)v$, so the question is if $v\in L^2(S^1)$ and $-\partial_x^2 v+W(x)v \in L^2(S^1)$, where $-\partial_x^2$ is distributional derivative, how to prove $v\in H^2(S^1)$?
If $W\in L^{\infty}(S^1)$, we get the conclusion by elliptic regularity, but how to deal the case of $W\in L^2(S^1)$?
