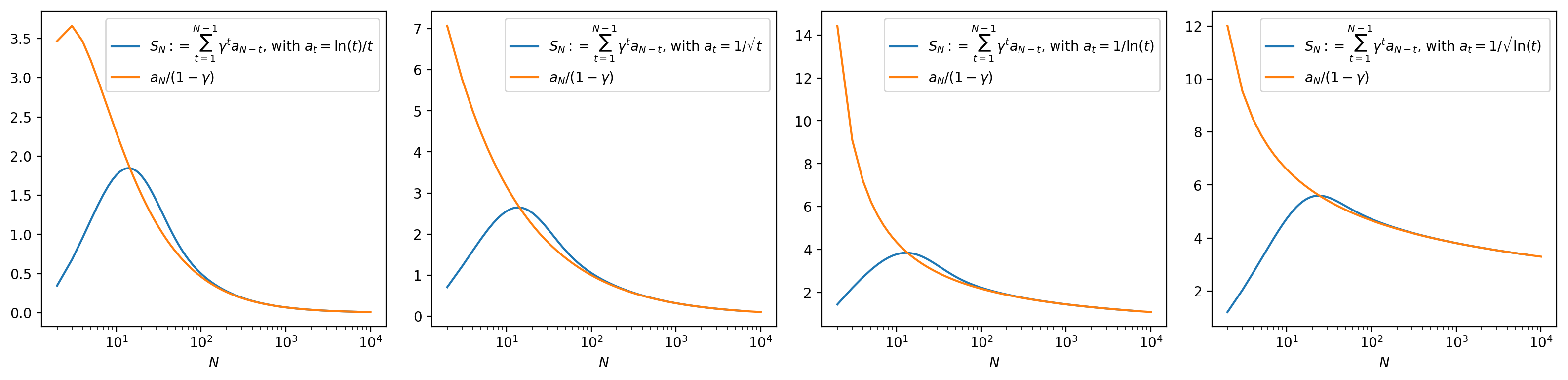
Given $\gamma \in [0, 1)$, an integer $N \ge 2$, and a decreasing null sequence of positive numbers $e_1,e_2,\ldots,e_t,\ldots$, I'm interested in estimating the sum $S_N := \sum_{t=1}^N\gamma^t e_{N-t}$.

Question

What is a good upper bound for $S_N$ for large $N$ ?

Observations

Empirically, I'm observing (by plotting graphs) that $S_N \sim \dfrac{e_N}{1-\gamma}$, but I'm not able to prove this in general. My experiments have been for $e_t=at^{-b}$ (with $a,b>0$), $e_t = \ln(t)/t$, $e_t=1/\ln(t)$, $e_t=1/\ln(t)^2$, etc.

The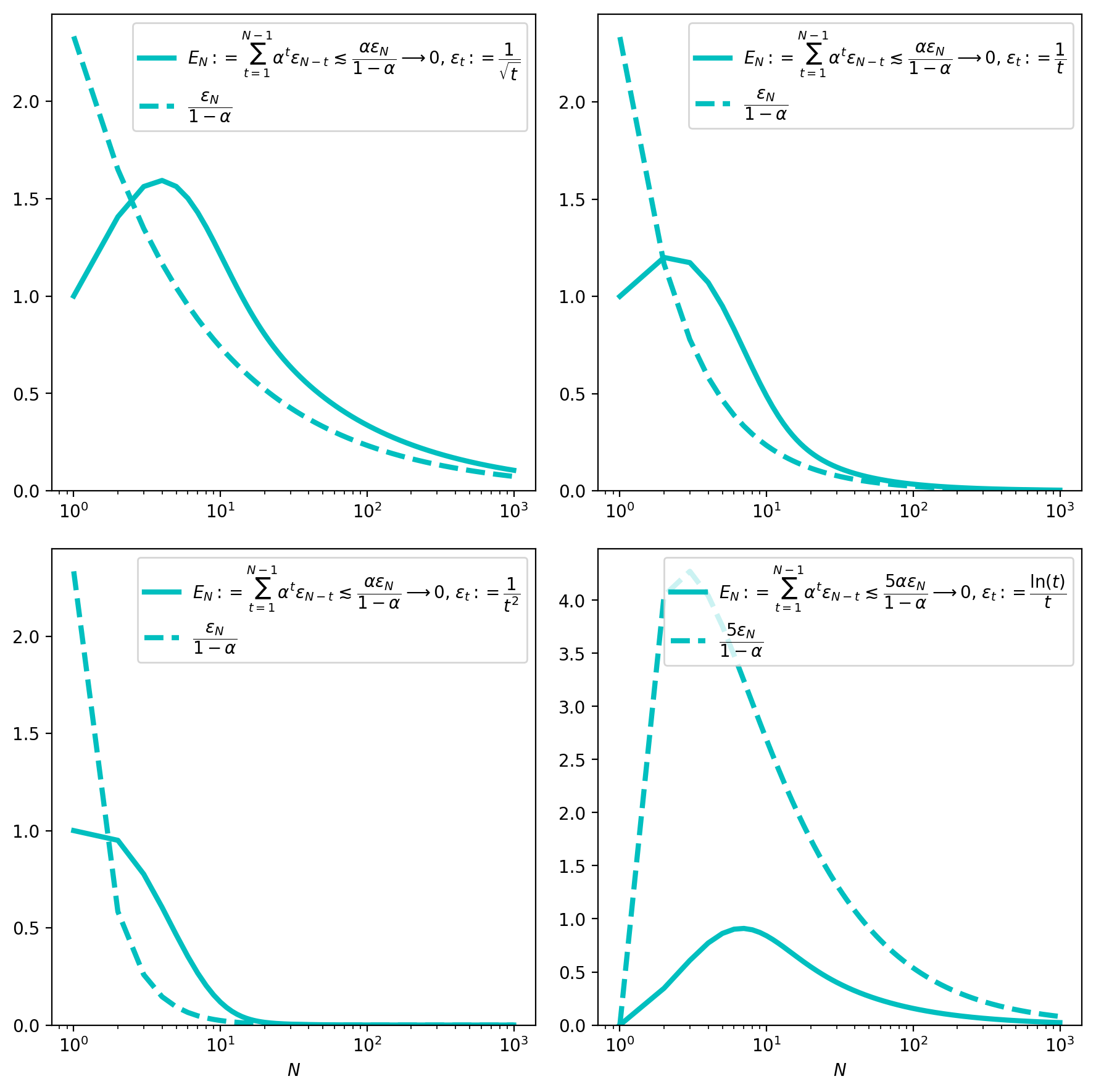 The case $e_t = at^{-b}$ can be established analytically. Indeed, a tedious computation reveals that $S_N \sim \frac{1}{1-\gamma}N^{-b} \sim \frac{1}{1-\gamma}e_N$.

Notes

• In my (abuse of notations), it's fine for $\sim$ to hide global multiplicative constants (e.g $e_1$, etc.).