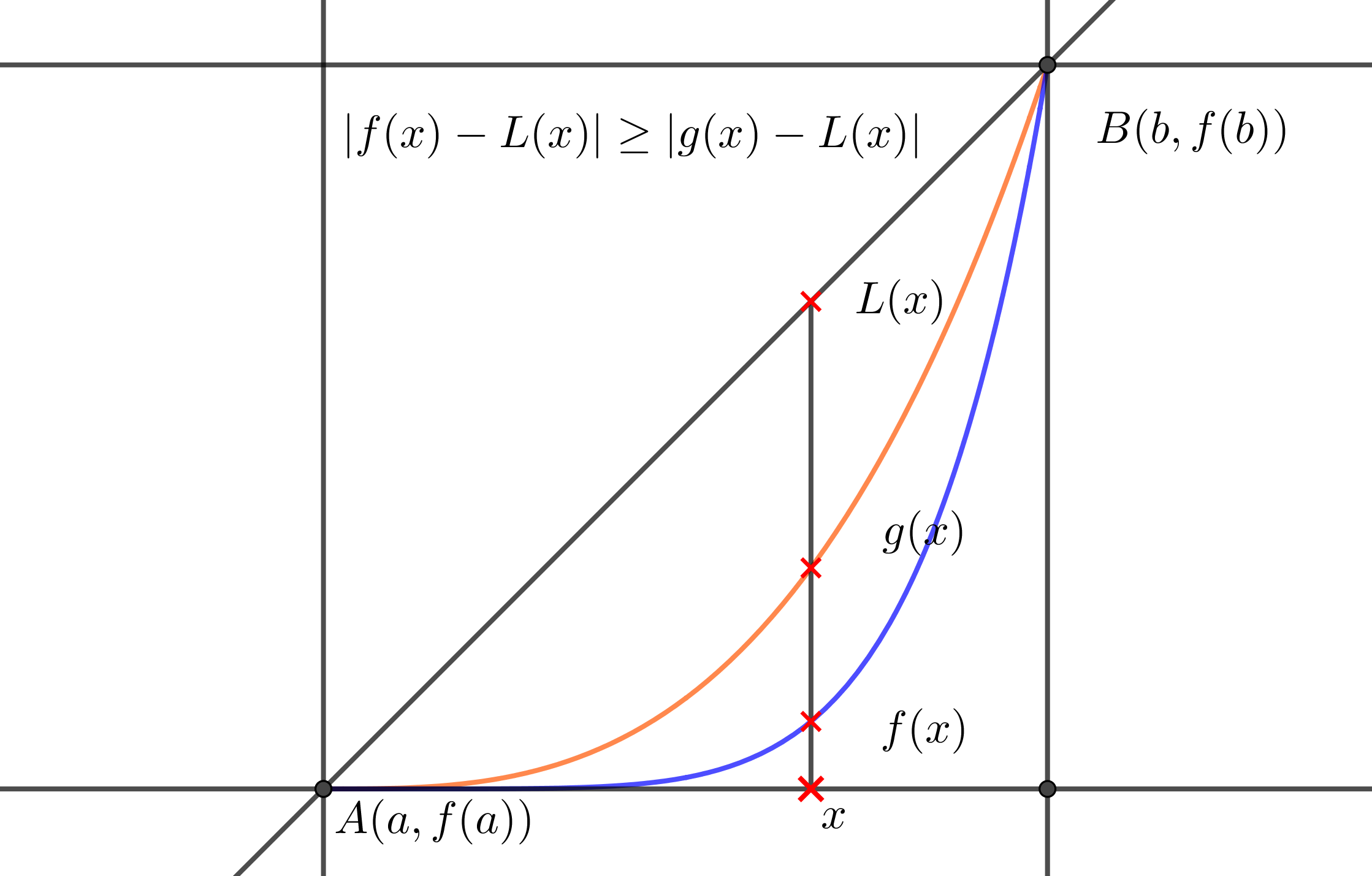
I am looking for a proof, reference, comment of an inequality as follows:
If $f(x)$ and $g(x)$ be two continuous derivative funcions in interval $[a, b]$. Such that:
$f(a)=g(a)$ and $f(b)=g(b)$
$($$f''(x) > 0$ or $f''(x) < 0$$)$ and $($$g''(x) > 0$ or $g''(x) < 0$$)$
$$|f(x)-\frac{f(b)-f(a)}{b-a}(x-a)+f(a)|\ge|g(x)-\frac{g(b)-g(a)}{b-a}(x-a)+g(a)|$$$$|f(x)-\frac{f(b)-f(a)}{b-a}(x-a)-f(a)|\ge|g(x)-\frac{g(b)-g(a)}{b-a}(x-a)-g(a)|$$
Then
$$\int_{a}^{b}{ \sqrt{1+f'(x)^2}}dx \ge \int_{a}^{b}{ \sqrt{1+g'(x)^2}}dx$$