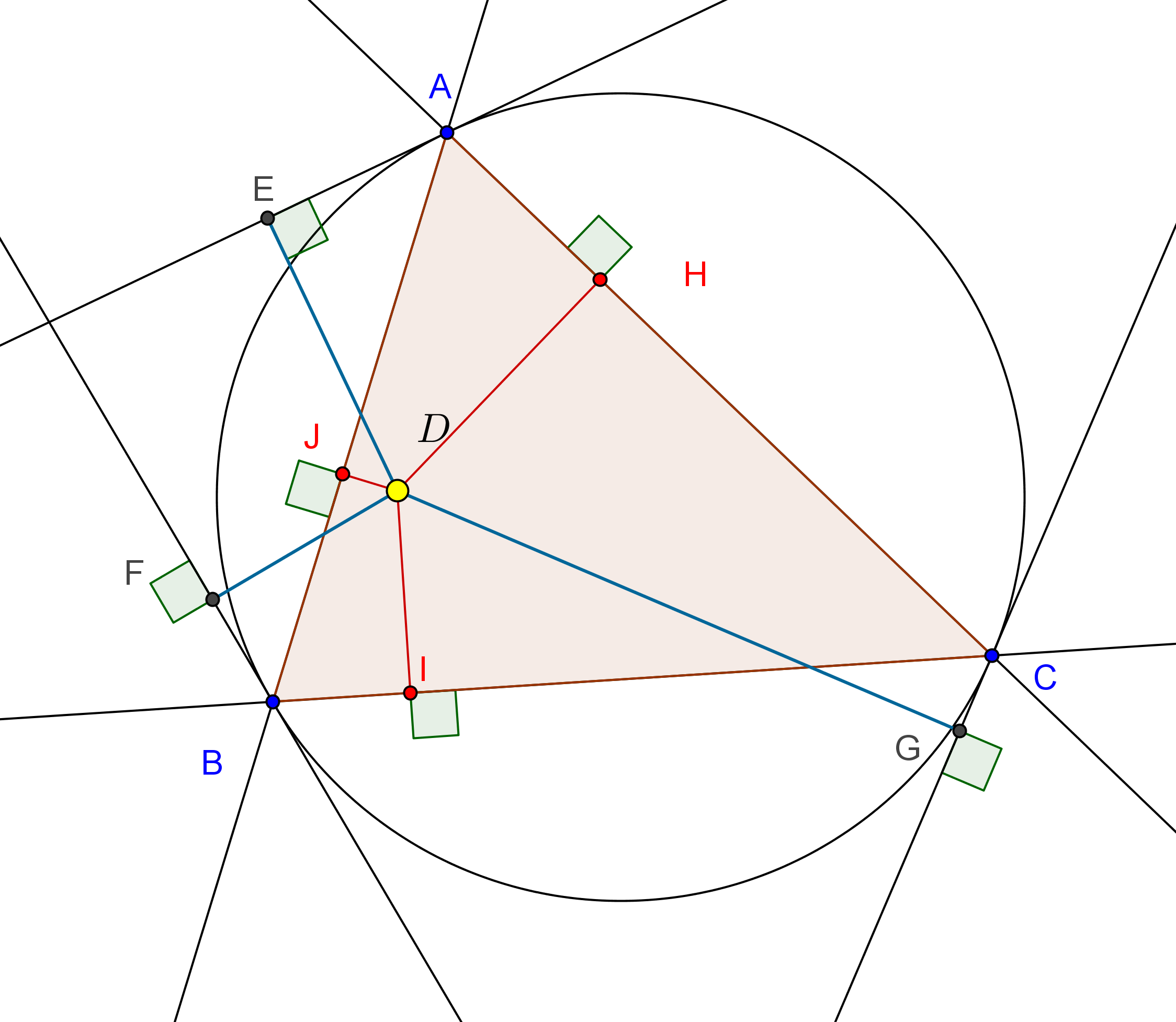
I proposed my conjecture, it is strengthened version of the Erdős–Mordell inequality as following:
Let $A_1A_2.....A_n$ be a cyclic polygon and $B_1B_2....B_n$ be the its tangential polygon. Let P$P$ be an arbitrary point inside of $A_1A_2.....A_n$. Let $D_i$$d_i$ be the distances from $P$ to $A_iA_{i+1}$, for $i=1,...,n$ and $A_{i+1}=A_1$, and $d_i$$D_i$ be the distances from $P$ to $B_iB_{i+1}$, for $i=1,...,n$ and $B_{i+1}=B_1$. Then show that:
$$\sum_{1}^{n}{ D_i} \ge sec{\frac{\pi}{n}}\sum_{1}^{n}{d_i} $$
Equality holds when $A_1A_2....A_n$ be the regular polygon.
A proof of the case polygon is a triangle in the paper A strengthened version of the Erdős-Mordell inequality", Forum Geometricorum, 16: 317–321, MR 3556993. I am looking for a proof of general case.