This is raised by a recent question occurring in combinatorial geometrya recent question occurring in combinatorial geometry.
It is about a sort of tractrix, but instead of a line, the pulling end moves along a circle of radius $r>\frac12$ (typically around $0.8$), starting with a chord if length 1. Imagine this chord horizontally on the bottom and take its center as the origine of a cartesian coordinate system. If the circle has center $(0,h)$, we have $h^2+\frac14=r^2$, so only one of $h$ and $r$ is needed to define the tractrix.
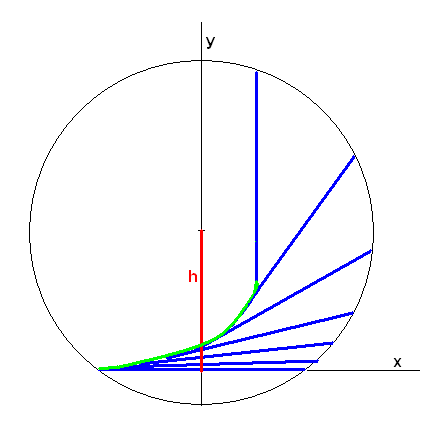
The tractrix is approximately the green line in the picture, where all blue segments (the tangents) have unit length.
Here is what I have got so far :
For a point $(x,y)$ on the tractrix where it has slope $f’$, we have 3 equations for the point $(u,v)$ where the tangent intersects the circle on the right:
$(i)\ \ \ \ v-y=(u-x)f’\ $ (equation of the tangent)
$(ii) \ \ \ u^2+(h-v)^2=r^2\ $ (intersection with the circle), equivalently $u^2+v^2-2hv-\frac14=0$.
$(iii)\ \ (u-x)^2+(v-y)^2=1\ $ (constant length of the tangent between tractrix and circle).
Eliminating $u$ and $v$ yields the following differential equation for the tractrix: $$x+\sqrt{1-s^2}=\sqrt{2h(y+s)-(y+s)^2-\frac14}$$ where $$s :=\frac{f'}{\sqrt{1+f’^2}}.$$ (Thus $s$ is the sine of the slope angle.)
I don’t think there is a closed form of the tractrix equation. But is there a way to determine at which point $(x,y)$ the tangent is vertical? I'd expect it to be a not-too-involved function of $h$.
Note that, unless $r$ is too small for reaching the vertical position at all, the tractrix will carry on winding beyond the vertical for a finite time. That is, under the assumption that at each moment, the segment is tangent to the tractrix at its (the segment's) endpoint. (Imagine $r$ a bit smaller than in the picture, such that the vertical tangent of the tractrix coincides with the $y$-axis. From there on, the top of the blue segment cannot move further to the left without "breaking the smoothness" of the tractrix. I think that for each $r$, the movement will eventually arrive at such a point, which we will naturally consider as the endpoint of the tractrix.)
But where is that point? I have no idea how far it can go if $r$ is big, but I don't think it will go further than becoming horizontal again. All this is easier to perceive if we keep $r$ constant and require the 'rotating' segment of length $\epsilon$ instead of unit length. So:
Where does the tractrix stop if $\epsilon\to0$?
