I want to understand why: From K.C Chang's book "Infinite Dimensional Morse Theory and Multiple Solution Problems":
if i have
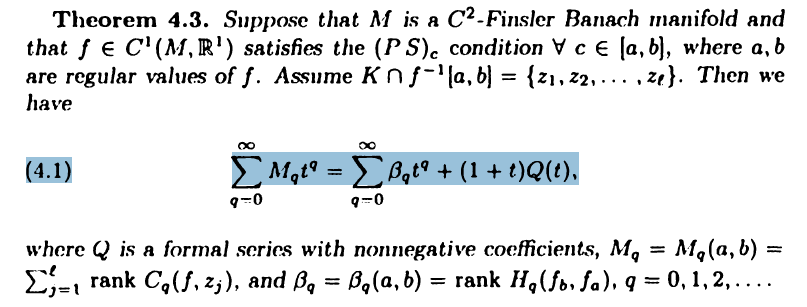
then $(4.1)$ is formal : it means that
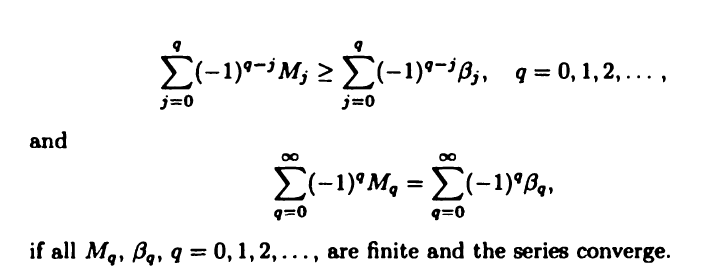
EDIT1: $(4.1)$ tel us that $\displaystyle\sum_{q=0}^{\infty} (M_q-\beta_q)t^q=(1+t)Q(t)$ Whene $t=-1$ we have directly that $\displaystyle\sum_{q=0}^{\infty}(-1)^q M_q=\displaystyle\sum_{q=0}^{\infty} (-1)^q \beta_q$
and wehave also that $\displaystyle\sum_{q=0}^{\infty}(-1)^q M_q\geq\displaystyle\sum_{q=0}^{\infty} (-1)^q \beta_q$ because $Q(t)$ has nonnegative coefficient
but how to obtain that $\displaystyle\sum_{j=0}^{q}(-1)^{q-j} M_j\geq\displaystyle\sum_{j=0}^{q} (-1)^{q-j} \beta_j$ ???
I ask this question on Mathematics stackex change but they don't answer me and they told me to ask it here
i need your help please help me
thank you
