Dear MOs,
Here is a calculus problem which bored me for sometime. Let $a>0$ and $b<0$ be fixed.Define the following function (EDIT: Following the comment by Barry Cipra, you may only consider the case where $a=1$)
$$ W_{a,b} (x)= e^{-2 b x}\left( \Phi^2\left(\frac{a b -x}{\sqrt{a}}\right)-\Phi\left(\frac{2 a b -x}{\sqrt{a}}\right)\right), $$
where
$$ \Phi(x): = \frac{1}{\sqrt{2\pi}}\int_{-\infty}^x e^{-y^2/2} d y = \frac{1}{2} \left(Erf\left(\frac{x}{\sqrt{2}}\right)+1\right). $$
The question is whether the following equation has only three zeors: $x=0$ and $x=\pm\infty$:
$$ W_{a,b}(x) = W_{a,b}(-x). $$
Plotting the function $W_{a,b}(x)$ suggests that the answer is right. But to find a proof seems quite hard.
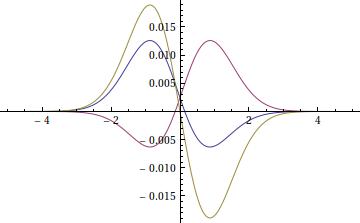
Here are some graphs of the functions: $W_{1,-1}(x)$ (the blue one), $W_{1,-1}(-x)$ (the red one), and $W_{1,-1}(x)-W_{1,-1}(-x)$ (the one crossing the origin).
Here is the original problem. Define
$$ E_{a,b}(x) = e^{-b x}\Phi\left(\frac{ab-x}{\sqrt{a}}\right)+e^{b x}\Phi\left(\frac{ab+x}{\sqrt{a}}\right). $$
We wish to prove that for $a>0$, $b<0$,
$$ E_{a,b}^2(x)\ge E_{a,2b}(x),\quad\text{for all $x\in R$.} $$
If one define
$$ F_{a,b}(x) =E_{a,b}^2(x)- E_{a,2b}(x), $$
then
$$ \frac{d F_{a,b}(x)}{d x} = -b \left( W_{a,b}(x) - W_{a,b}(-x)\right). $$
Hence, this problem reduces to the above question.
Thank you very much for any suggestions!
Anand