If you take the diagram of the Reidemeister 3 move and "shortcircuit" two ends, you get (click https://i.sstatic.net/gfOKy.jpg if Imgur hotlink doesn't work): 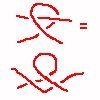 I have circumstantial evidence that this weaker version is actually equivalent to R3. (Only in a computational sense! My hypothesis: If A and B are two diagrams of the same knot, while it might not be actually possible to go from A to B by applying weak R3 moves (+R2+R1, of course), the assumption that weak R3 holds forces invariant(A)=invariant(B) for any Lie group derived invariant. Likewise, in Kauffmans abstract tensor framework, just assume weak R3, solve and get the rest of the Yang-Baxter equation for free.) Thus: Is there work on "alternative moves"? Can you construct a counterexample? (I.e. an pseudo-invariant which is constant under weak R3+R2+R1, but not under R3? The example must have a skein equation, though.)