50
$\begingroup$

Is there a nontrivial link in a big solid torus that is trivial in the ambient Euclidean space such that each circle is unknot and has a sufficiently small length?

It is motivated by a question that bothers me from my childhood:

Is it possible to wrap a suitcase with hair ties without tying them together?

enter image description here

Comments

  • The answer of Larsen Linov is accepted, but it remains to prove formally that the link meets the conditions. The latter is equivalent to nontriviallity of the following link; the example of Larsen Linov (or a similar one) can be obtained by stating that one of circles is a meridian of the solid torus.

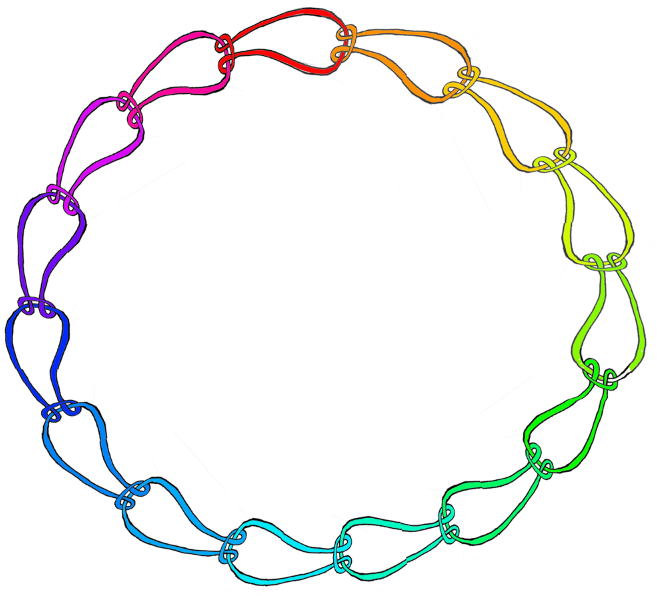
picture from here https://math.stackexchange.com/questions/4338772/is-this-object-a-simpler-brunnian-rubberband-loop-than-those-studied

  • Another question: is it possible to do the same with large rotational symmetry?
Improve this question
$\endgroup$
6
  • 5
    $\begingroup$ I don't have an answer, but the term "rubberband Brunnian link" seems relevant. $\endgroup$
    – Timothy Chow
    Commented Jun 7, 2022 at 22:38
  • 4
    $\begingroup$ If this were possible then every nine-year old with a rubber band loom would know about it… $\endgroup$
    – Sam Nead
    Commented Jun 8, 2022 at 2:14
  • 4
    $\begingroup$ Here is a geometric proof. Let $L_n$ be the rainbow link you drew. Then $L_n$ is a "meridional" Dehn filling of a cyclic $n$-fold cover of a two-component ten-crossing hyperbolic link (called L10n36 by SnapPy). These two components are, respectively, the unknot and the connect sum of a left and right trefoil. Since the meridional slope of the unknotted component grows linearly with $n$ we may apply Thurston's hyperbolic Dehn filing and deduce that all but finitely many of the $L_n$ are hyperbolic. $\endgroup$
    – Sam Nead
    Commented Jul 11, 2022 at 8:45
  • 2
    $\begingroup$ Nicest question of the year!! Isn't there a badge for that? i think I'll do this question in all my topology-based dissemination :) $\endgroup$
    – Andrea Marino
    Commented Jul 11, 2022 at 21:31
  • 6
    $\begingroup$ This question now has a great YouTube video by Henry Segerman, Sabetta Matsumoto, and Saul Schleimer at youtu.be/Cyhqc8l03GE . $\endgroup$
    – Mark S.
    Commented Jun 29, 2023 at 0:41

2 Answers 2

Reset to default
28
$\begingroup$

This configuration should work:

hair ties solution

Edit (to provide credit/context): Michael Freedman's solution (see Ian Agol's post) is the original one. Ian directed me to this problem and gave me the hint that Michael had already confirmed it was possible.

Improve this answer
$\endgroup$
4
  • 8
    $\begingroup$ I assembled this - it works perfectly as a bracelet, thanks! $\endgroup$
    – isaacg
    Commented Jul 11, 2022 at 3:07
  • 3
    $\begingroup$ I am sure that it works, but can you prove it? $\endgroup$
    – Anton Petrunin
    Commented Jul 11, 2022 at 6:59
  • 10
    $\begingroup$ To complete the answer, you need to make a three-minute YouTube instructional video titled "How to Make a Loom Band Bracelet Using Topology Instead of a Clip". $\endgroup$
    – Sam Nead
    Commented Jul 11, 2022 at 9:19
  • 2
    $\begingroup$ @SamNead youtube.com/watch?v=Cyhqc8l03GE $\endgroup$
    – Anton Petrunin
    Commented Jun 29, 2023 at 20:54
11
$\begingroup$

I shared this question with Michael Freedman who came up with a solution similar to Larsen’s and asked me to post on his behalf.

Michael Freedman's solution

He made a physical realization too.

hair ties

I’ll address Anton’s question of how to prove that this is not the unlink. There is a general method of Haken and Waldhausen to show that manifolds are Haken, and hence have incompressible boundary (in which case they cannot have an unknot component). Exhibiting a simple hierarchy can prove that a manifold is Haken. One can show that the handlebody Freedman embeds has incompressible complement in the solid torus by exhibiting a simple hierarchy. The first stage is annuli going around the loops. After that it becomes compressible, and the next stage of the hierarchy is a compressing disk. The last stage is torus × I, and one can finish off with a hierarchy for this with annuli and disks. Inside the handlebody (at the top of the diagram), the link is also essential. There are a variety of hierarchies one could use here: first use 4-punctured disks to break it up into pieces of finitely many types (most of which look like the box in Larsen’s picture), then analyze each of these finitely many pieces. Hopefully this gives a flavor of how these certificates behave.

Addendum: Okay, I see what Anton is saying now. Larsen’s (or Mike’s) configuration is equivalent to:

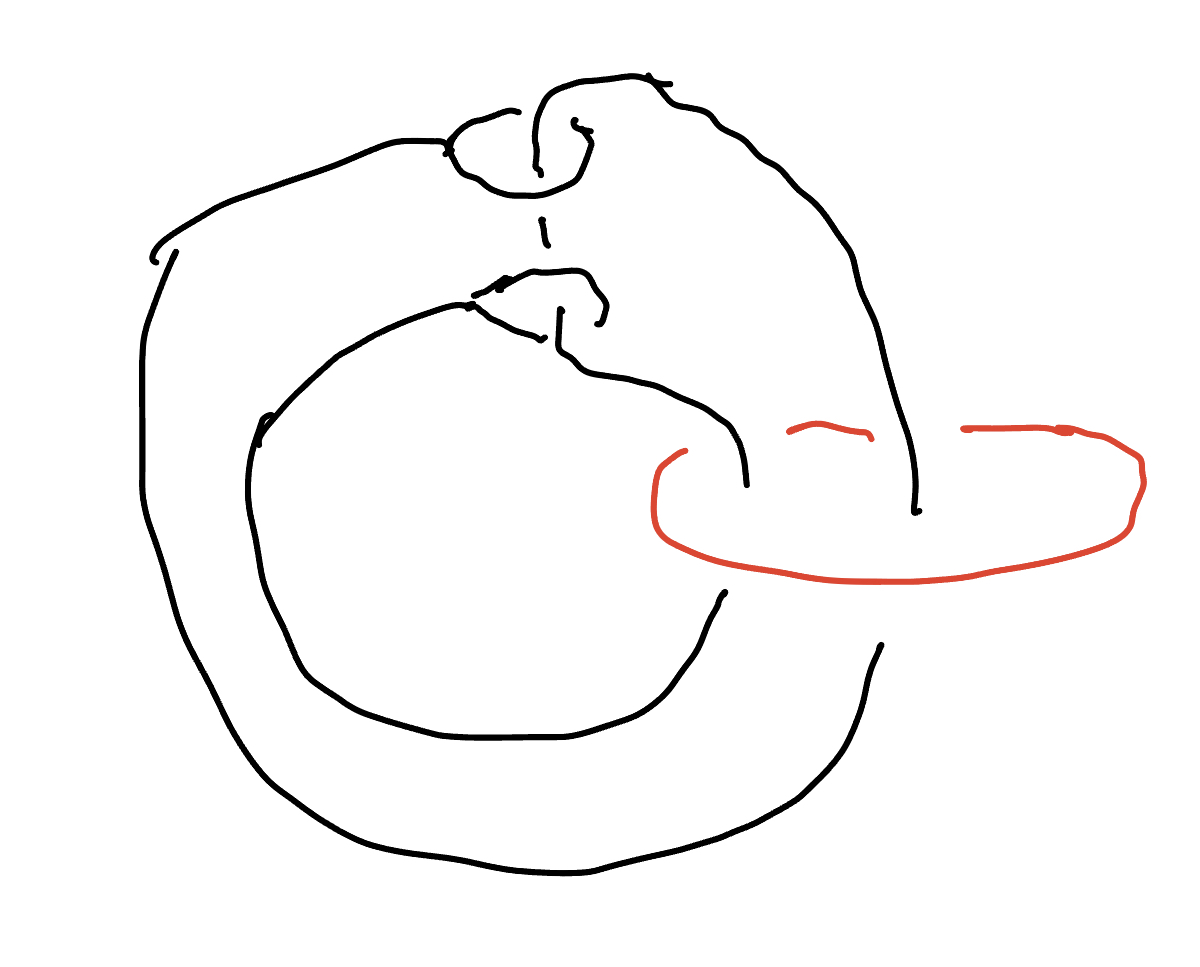
Equivalent configuration

This can be deformed to:

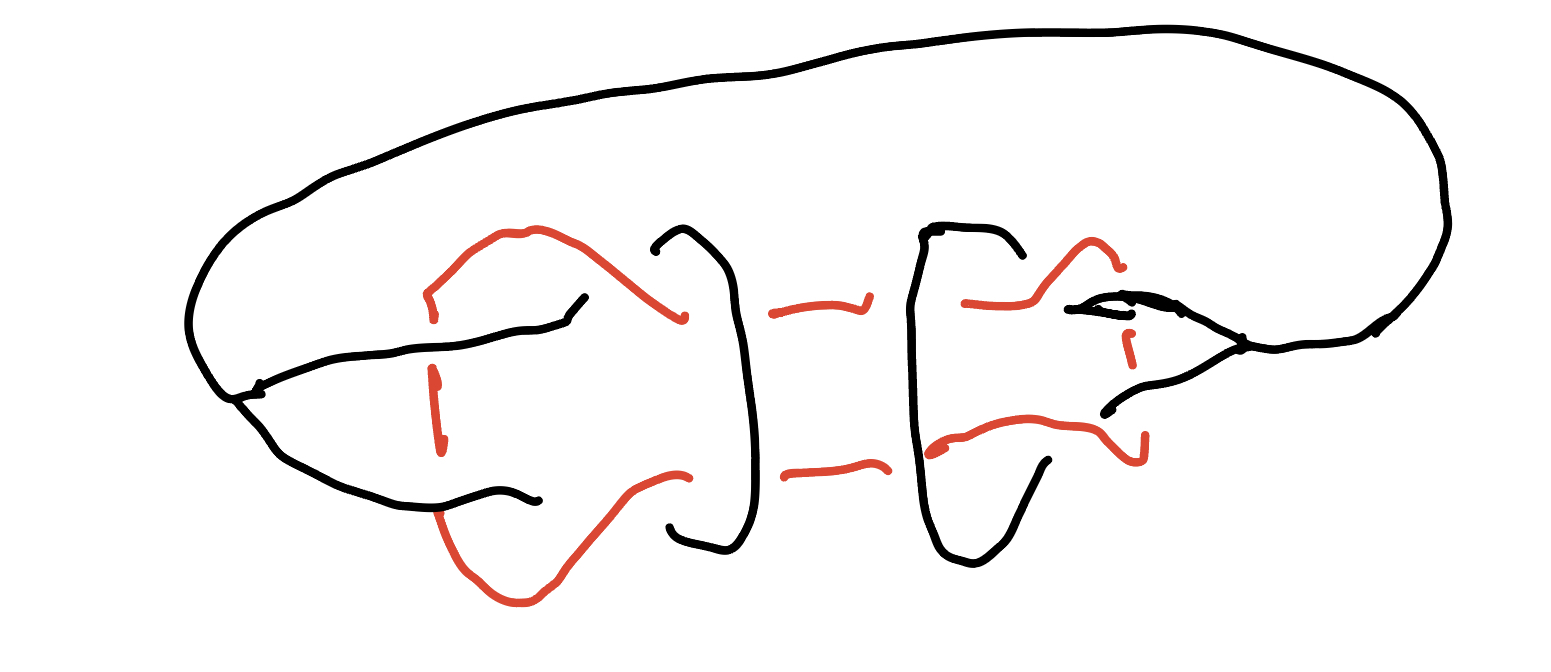
Deformed configuration

Inserting the Brunnian chain pattern into here gives the link shown in Anton’s comment, which Sam Nead proves is a non-trivial link in the comments.

Improve this answer
$\endgroup$
12
  • 3
    $\begingroup$ It might be more helpful to later readers to instead write “which Sam Nead proves”. :) $\endgroup$
    – Sam Nead
    Commented Jul 11, 2022 at 18:45
  • 2
    $\begingroup$ @LSpice thanks for this, we were probably editing at the same time, but you finished first. Go ahead and re-edit if you like, or I’ll have a look when I get a chance. $\endgroup$
    – Ian Agol
    Commented Jul 11, 2022 at 20:22
  • 2
    $\begingroup$ I have re-made the edit, and made the Saul → Sam Nead change, as well as linking the relevant proof. You reference "the link shown in Anton's comment", but I couldn't find that one, so didn't link it (different meanings of 'link' 😄). $\endgroup$
    – LSpice
    Commented Jul 11, 2022 at 20:33
  • 2
    $\begingroup$ @LSpice In the comment he added in his answer, he has a picture of a rainbow colored link. I’m proving that Larsen’s example is isotopic to his (which clearly Anton already knew). $\endgroup$
    – Ian Agol
    Commented Jul 11, 2022 at 20:35
  • 1
    $\begingroup$ Not being familiar with this method of Haken and Waldhausen, it's not clear to me: what is the minimal number of components of the link such that this works? Of course one component doesn't work -- does it work with two components? $\endgroup$
    – Tim Campion
    Commented Jul 11, 2022 at 21:06

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .