Does there exist a continuous map $f:\mathbb{R}\rightarrow \mathbb{R}$ such that the forward orbit of 0 is dense in $\mathbb{R}$?
-
4$\begingroup$ Existence of a point with the dense orbit is one of the definitions of topological transitivity. To see some examples of such maps on $\mathbb{R}$, google "transitive map on R" $\endgroup$– erzCommented Apr 29, 2020 at 2:03
-
$\begingroup$ I found a topologically transitive map on \mathbb{R} with backward orbit of every point dense on \mathbb{R}, but not the forward orbits.(Reference:- researchgate.net/publication/…) $\endgroup$– yogamatCommented Apr 29, 2020 at 3:35
-
2$\begingroup$ @yogamat therefore the paper you link, + the one I provide (Proposition 2) seems to answer your question, except that the proof doesn't tell you which point has a dense forward orbit: it just tells you that every point in a dense $G_\delta$-dense subset $B$ has a dense orbit. This set $B$ is explicit but exhibiting a point in it doesn't seem easy in such an example as the piecewise affine map from your link. $\endgroup$– YCorCommented Apr 29, 2020 at 5:20
-
1$\begingroup$ mathoverflow.net/a/363010/53155 $\endgroup$– erzCommented Jun 14, 2020 at 4:42
-
5$\begingroup$ A more challenging question: does there exist a Lipschitz map on ${\bf R}$ with dense orbit? $\endgroup$– Terry TaoCommented Sep 27, 2020 at 3:19
2 Answers
As in this previous answer of mine, emulating random walks works pretty well for this kind of question.
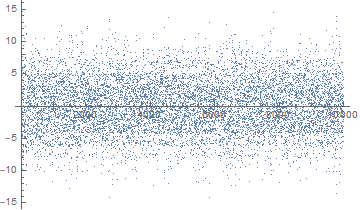
Consider the map $T : \mathbb{S}_1 \to \mathbb{S}_1$ defined by
$$T(x) = 5x [1] \ \text{ if } \ 1/5 \leq x < 4/5,$$ $$T(x) = -5x [1] \ \text{ otherwise.}$$
Its graph is as follows:
The map $T$ is continuous, preserves the Lebesgue measure, is ergodic, and much more.
Now, let me introduce the $\mathbb{Z}$-extension $\widetilde{T} : \mathbb{S}_1 \times \mathbb{Z} \to \mathbb{S}_1 \times \mathbb{Z}$ of $T$ defined by
$$\widetilde{T} (x, p) := (T(x), p+F(x)),$$
where $F(x) = -1$ for $x \in [0,2/5)$, then $F(x) = 0$ for $x \in [2/5,3/5)$ and $F(x) = +1$ for $x \in [3/5,1)$. Note that $\widetilde{T}$ preserves the uniform ($\sigma$-finite) measure on $\mathbb{S}_1 \times \mathbb{Z}$.
The second coordinate of $\widetilde{T}^n (x, p)$ is $p+S_n F(x) := p+\sum_{k=0}^{n-1} F (T^k (x))$. Under the Lebesgue measure on $\mathbb{S}_1$, the sequence $(F \circ T^k)_{k \geq 0}$ is a sequence of i.i.d. random variables of symmetric distribution $2/5\cdot \delta_{-1} + 1/5 \cdot \delta_0+ 2/5\cdot \delta_{+1}$, so that the process $(S_n F)_{n \geq 0}$ is ergodic and recurrent.
A bit more work (but not that much, given the simplicity of the model) gives that $\widetilde{T}$ is ergodic and recurrent for the uniform measure on $\mathbb{S}_1 \times \mathbb{Z}$. This is very much folklore, although I have to admit it can get annoying to pinpoint the best reference and fill the gaps. As a consequence, almost every point has a dense orbit.
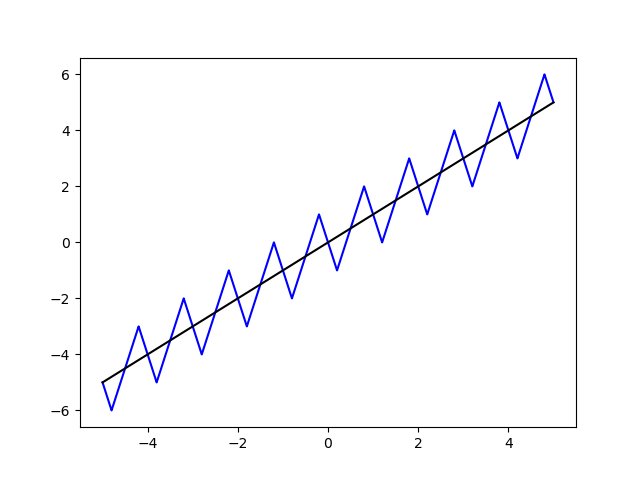
Now, everything is on $\mathbb{S}_1 \times \mathbb{Z}$; however, is we identify $\mathbb{S}_1$ with $[0,1)$ and then $\mathbb{S}_1 \times \mathbb{Z}$ with $\mathbb{R}$, we get a map $S$ from $\mathbb{R}$ to $\mathbb{R}$. The specific choice of $T$ gives that $S$ is continuous, actually 5-Lipschitz, with a sawtooth-like graph (in black, the line with equation $y=x$):
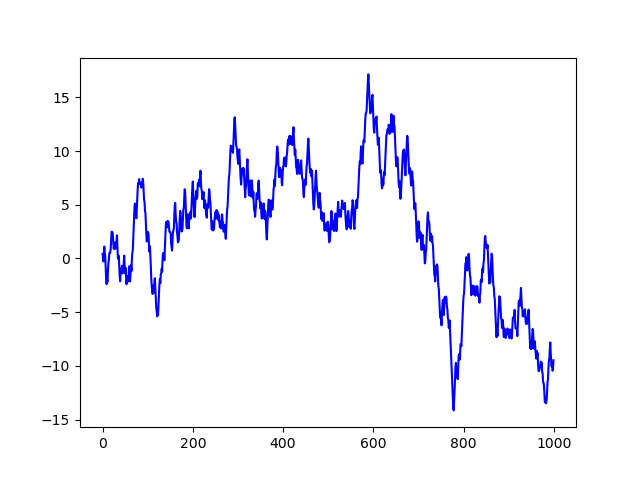
Again, Lebesgue-almost every point has a dense orbit. Here is the picture of an orbit from a random (uniform in $[0,1]$) starting point:
The orbit of $0$ is not dense, as it is a fixed point; however, conjugating by a Lebesgue-generic translation gives the map we want.
The same construction work on $\mathbb{R}^2$ (just use $S \times S$), where Lebesgue almost every orbit will be dense. Things get more annoying in higher dimension, since the random walks are no longer recurrent. This can be solved by getting a tweak $R$ of $S$ favouring orbits recurring quickly to zero, ensuring that $R$ preserves a unique absolutely continuous invariant measure, with respect to which it is mixing; then $(R, R, \ldots, R)$ is mixing with respect to the product measure on $\mathbb{R}^n$, and from there topologically mixing.
-
$\begingroup$ @Matt F.: No. If $x < 1/5$, then $T(x) = 1-5x$. Going back to the real number, we get $S(x) =1-5\{x\}+ \lfloor x \rfloor + F(\{x\}) = \lfloor x \rfloor + 1-5\{x\}-1 = \lfloor x \rfloor -5\{x\}$ whenever $\{x\} < 1/5$, so the sequence $(x_n)$ can decrease. $\endgroup$ Commented Sep 22, 2021 at 22:25
-
$\begingroup$ Basically, the graph of this function starts from $(0,0)$, goes down to $(1/5, -1)$, goes up to $(4,/5, 2)$, goes down to $(6/5, 0)$, goes up to $(9/5, 3)$, etc. I will post a graph tomorrow to make the construction more intuitive (because it really is) ; it's a situation where a picture is worth a thousand words. $\endgroup$ Commented Sep 22, 2021 at 22:28
-
$\begingroup$ @Matt F.: The images are now there. $\endgroup$ Commented Sep 23, 2021 at 9:21
-
$\begingroup$ Now I agree that this works from a generic starting point, and can be tweaked to (apparently) work from $0$. I would describe your construction as: Let $$S_0(x) = \lfloor x \rfloor + \min(5(x- \lfloor x \rfloor), 6 - 5(x - \lfloor x \rfloor)),$$ $$S(x)=S_0(x-\frac15)-1.$$ Then it is easy to see that both $S_0$ and $S$ also define maps on $\mathbb{R}/\mathbb{N}$. Furthermore $$S(x)=S_0(x-\frac13)-\frac{13}{15}$$ seems to give a dense forward orbit of $0$, and (answering @TerryTao's question) with a Lipschitz function. $\endgroup$– user44143Commented Sep 24, 2021 at 17:05
Here is a possibility, inspired by D. Thomine's answer (without deciding whether the answers are the same or whether either is right). Let
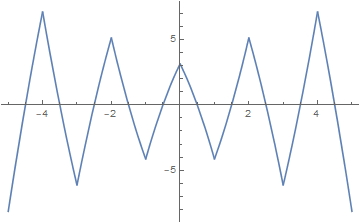
$$S(x)=(-1)^{\lfloor x\rfloor}(\pi+|x|)(1-2x+2\lfloor x \rfloor))$$
Then $S$ looks like
and the first $10000$ iterates of $0$ look like
That graph goes from $-14.2$ to $14.6$, and after a million iterates it goes from $-26.0$ to $26.6$. The first four moments and the extremes of these iterates are roughly what one would expect from a normal distribution with standard deviation $4$. So I suspect that the infinite set of iterates is roughly normal and dense in $\mathbb{R}$; perhaps someone will see how to use ergodic theory to prove that.
-
-
1$\begingroup$ It now looks to me like this is different from D. Thomine's answer, and his is probably right and easier to prove correct. $\endgroup$– user44143Commented Sep 24, 2021 at 16:25