Here is a possibility, inspired by D. Thomine's answer (without deciding whether the answers are the same or whether either is right). Let
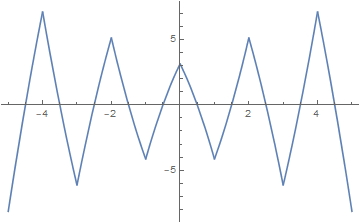
$$S(x)=(-1)^{\lfloor x\rfloor}(\pi+|x|)(1-2x+2\lfloor x \rfloor))$$
Then $S$ looks like
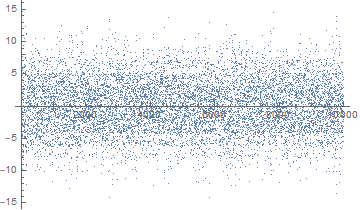
and the first $10000$ iterates of $0$ look like
That graph goes from $-14.2$ to $14.6$, and after a million iterates it goes from $-26.0$ to $26.6$. The first four moments and the extremes of these iterates are roughly what one would expect from a normal distribution with standard deviation $4$. So I suspect that the infinite set of iterates is roughly normal and dense in $\mathbb{R}$; perhaps someone will see how to use ergodic theory to prove that.