13
$\begingroup$

Imagine $n$ $z$-vertical sticks uniformly spaced around a unit-radius circle in the $xy$-plane. At $t{=}0$, each is randomly $\epsilon$-perturbed from the vertical, and they fall under the influence of gravity. Will some sticks form a "teepee" suspended above the $xy$-plane?

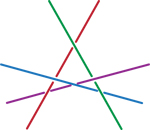
Let us assume the sticks are one-dimensional segments of height $h$, perhaps $h{=}2$ so they span the diameter, and that their base points are pinned to the plane via universal joints. It seems possible that a subset of sticks could fall to form a weaving with a cyclic on-top-of graph, as illustrated below.
Woven lines
Assuming a sufficient coefficient of friction $\mu$ between pairs of sticks, it seems conceivable that such a structure would not collapse to the plane.

What is your intuition here? For sufficiently large $n$, and adequate $h$ and $\mu$, would some sticks form a woven structure above the plane? Or would all sticks ultimately flatten to the plane? If the latter, are there natural conditions that would lead to formation of a "teepee"? I am less interested in probability calculations than in a qualitative assessment.

I've tagged this 'recreational' because it is only a peripheral spinoff of my research.

Addendum. It is now clear, from the comments of Scott Morrison and Rahul Narain, that the random perturbations should be random both in direction and magnitude. Otherwise, as Scott incisively observed, the sticks, with high probability, all fall without touching one another until they reach the plane (which to me is already rather remarkable!).

Improve this question
$\endgroup$

4 Answers 4

Reset to default
4
$\begingroup$

Something doesn't seem right here. Let's ignore the 'coefficient of friction' and just make the sticks out of caramel: if they even touch they glom together. Nevertheless, everything is always flat!

If sticks ever glom, there's a pair of sticks that glom first. However, if you think about a pair of sticks falling in this way (I'm assuming $\epsilon$ is small, so all sticks make the same angle to the horizontal at any moment before the first glom), it's clear that they only actually make contact with each other at the instant they hit the ground. (The only other possibility is having the tips collide, which almost surely never happens.)

Perhaps you can make the sticks thicker and have something happen, but that seems extremely complicated.

Improve this answer
$\endgroup$
4
  • $\begingroup$ @Scott: Center the unit-radius circle on (0,0,0). Suppose the stick whose base is (1,0,0) falls 45 deg westward, and the stick whose base is (0,-1,0) falls 45 deg northward. Then if they are long enough (say,$h{=}2$), they intersect at (0,0,1). So it seems contact can occur prior to reaching the plane? $\endgroup$
    – Joseph O'Rourke
    Commented Jun 27, 2010 at 16:54
  • $\begingroup$ Oh, I see, in my example, the tips would hit first! Hmmm... So it appears that the random perturbations would need to be random in both direction and magnitude, as suggested by Rahul. Indeed it makes physical sense that they would not all fall synchronously. $\endgroup$
    – Joseph O'Rourke
    Commented Jun 27, 2010 at 18:08
  • $\begingroup$ Doesn't this argument presume that all the perturbations result in exactly the same angle from the vertical? Is that what was intended in the question? $\endgroup$
    – Joel David Hamkins
    Commented Jun 27, 2010 at 19:28
  • $\begingroup$ @Joel: Yes. I was not clear on this issue, because I did not realize that it had the effect of potentially trivializing the problem. My fault! But allowing truly random perturbations, all remains unclear (to me!). $\endgroup$
    – Joseph O'Rourke
    Commented Jun 27, 2010 at 21:18
4
$\begingroup$

Couldn't accomplish this in a comment to Rahul's #1, but here is a physical realization of my drawing with colored pencils. Compare:

Line drawing Physical realisation with pencils

The pencils are in contact, but the lead inside could serve as lines in space without contacts. I don't know how to reconcile this with your quote from Whiteley….

Improve this answer
$\endgroup$
3
  • $\begingroup$ Ah, sorry, I see now that your diagram is not the same as Whiteley's. In Whiteley's diagram, the cyan and purple pencils go under and over the red one respectively. $\endgroup$
    – user6979
    Commented Jun 28, 2010 at 15:08
  • 4
    $\begingroup$ Whew! Mathematics is consistent with reality! :-) $\endgroup$
    – Joseph O'Rourke
    Commented Jun 28, 2010 at 15:34
  • 1
    $\begingroup$ I note that you have to use cellophane tape to keep the pencils in contact with each other without slippage as their coefficient of friction $\mu$ is probably too low. Tensegrity would also require tension with wires connecting the endpoints of the pencils. $\endgroup$
    – sleepless in beantown
    Commented Sep 1, 2010 at 9:16
3
$\begingroup$

As Scott pointed out, sticks of zero width will necessarily all fall to the plane. If they are considered to be of extremely small thickness, even in an unwoven pile only the bottom stick(s) will be touching the ground. So a weave can really only be characterized as a cycle in the on-top-of graph.

If you allow varying the magnitudes of perturbation, then a stick can be made to stay up for an arbitrarily long time after other sticks have fallen. If, on the other hand, we simplify the problem by requiring the same magnitude of perturbation for all sticks, then the sticks fall in unison, such that at any time they all make the same angle with the horizontal.

In this case the problem can be reduced to its two-dimensional projection: Take $n$ points $p_1, \ldots, p_n$ on a unit circle, and draw a line segment $\ell_i$ of length $h$ in a random direction from each point. Segment $\ell_i$ is "on top of" $\ell_j$ if they intersect and the intersection point is farther from $p_i$ than from $p_j$. Is there a cycle in the on-top-of graph?

I had many conjectures based on this formulation, but I'm afraid I was not able to prove any of them.

Addendum addressing your updated question:

  1. Your example diagram is impossible to achieve with straight sticks, as Whiteley points out: "there is no pattern of lines in 3-space, without contacts, which projects to such a picture."

  2. If the sticks are of zero width, then regardless of the magnitude of perturbations they will all fall to the plane. This is because any configuration can be squashed linearly in z to send it closer to the plane while preserving the straightness of the sticks and the topology of the configuration.

  3. If the sticks are of nonzero width, a subset of sticks will be suspended above the xy plane without external support iff they form a cycle in the on-top-of graph. (Proof: if they form a cycle, no stick can fall to the xy plane as its predecessor would have to be below it; if there is no cycle, then there is a bottommost stick, which will not be suspended.) Such cycles can occur even if all sticks fall simultaneously (e.g. send them all slightly clockwise of the center, so that they fall to form a ruling of a hyperboloid of one sheet), so restricting the magnitude of perturbations simplifies the problem without trivializing it.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ #1 is not quite correct, as Joseph's comment shows. The example diagram here is realizable, but is not rigid in Whiteley's sense; Whiteley's Fig. 1A is subtly different, making it rigid but only achievable with bent sticks. This is a necessary condition for rigidity in Whiteley's sense, as he is interested in weavings that lie rigidly in a plane without having to pin any endpoints. $\endgroup$
    – user6979
    Commented Jun 28, 2010 at 15:54
  • $\begingroup$ @JosephO'Rourke's comment/answer referenced above, I think. $\endgroup$
    – LSpice
    Commented Jul 30, 2022 at 2:09
2
$\begingroup$

There is work by Walter Whiteley

and Robert Connelly

which though not dealing with probabilistic matters may be of interest or of use.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thanks for the references! The Connelly paper is primarily focused on tensegrities, but the Whiteley paper, "Rigidty and polarity," is quite apropos (at least from the abstract and the first page accessible without purchase). In fact, Whiteley's Fig.1 coincides with my drawing. He calls it the smallest stable or rigid configuration. $\endgroup$
    – Joseph O'Rourke
    Commented Jun 27, 2010 at 13:40

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .