There is a classic (and open) problem asking whether every polyhedron can be unfolded to give a non-overlapping net. The converse problem has been studied asking which polygons can be folded in some manner to give a polyhedron. For an overview of both problems and related discussion see:
Erik D. Demaine and Joseph O'Rourke Geometric Folding Algorithms: Linkages, Origami, Polyhedra Cambridge University Press, July 2007. ISBN 978-0-521-85757-4
Question
I want to ask about a more direct converse. Given a net of polygons connected at their edges when can they fold to form a polyhedron?
Example
As an example take the two nets shown below. By identifying the edges as shown by colour both satisfy the topological constraints to be sphere.
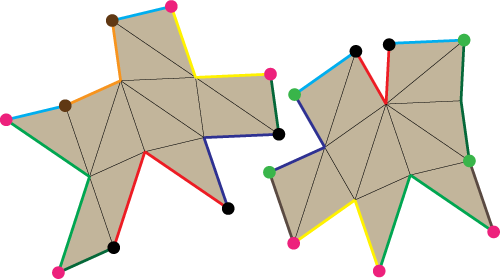
Only one, however, will fold to give a polyhedron:
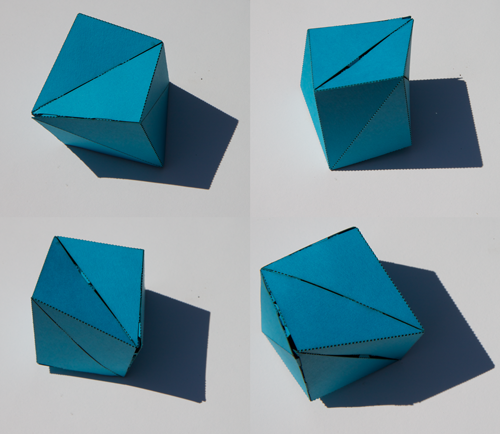
Personal Motivation
My motivation is to find visually appealing, simple, but non-symmetric, polyhedra. I have used equilateral triangles but would like to play with other shapes. General sufficient conditions would, therefore, be very interesting to me.
