Let $P$ be a convex polytope in Euclidean space $\mathbf{R}^3$ and $\Gamma$ be a closed curve which passes through all vertices of $P$. How small can the length $L$ of $\Gamma$ be? More specifically, if $A$ denotes the area of the boundary of $P$, can one show that $$ L^2\geq 2\pi A? $$ Here equality would hold only in the limiting case where $\Gamma$ converges to a circle in the plane, and $P$ becomes degenerate as its boundary collapses to a doubled disk. Thus this problem might be thought of as an extension of the isoperimetric inequality for planar curves to curves in space.

Note that the shortest curve $\Gamma$ for a given polytope $P$ needs not lie on the boundary of $P$. For instance consider a short wide octahedron.

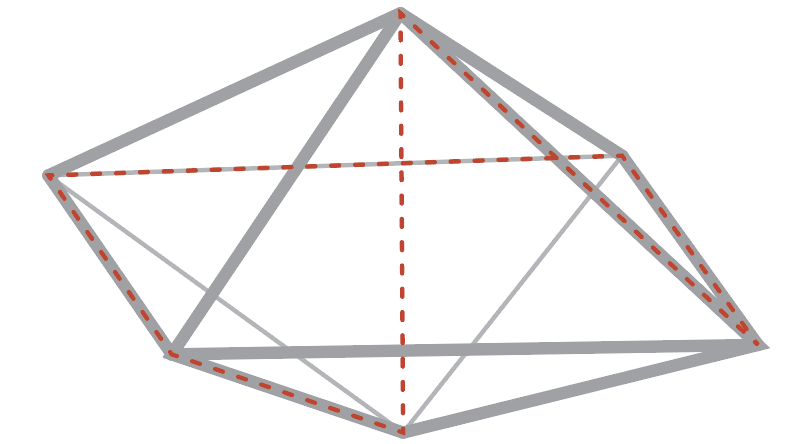
The above problem is the discrete version of a more general question: Among all closed curves of a given length in $\mathbf{R}^3$, which one maximizes the surface area of its convex hull? Of course if the above inequality is established for polygonal curves, then the general case follows.

The earliest reference to this problem seems to be in the book of Croft-Falconer-Guy, p. 38 (I would be interested to know if there is an earlier reference). A few years later it was discussed in a survey article of Zalgaller, who noted that the solution is easy if the curve lies on the boundary of the convex hull, in which case we might apply Weil's isoperimetric inequality for surfaces of nonpositive curvature. The analogous problem for the volume of the convex hull is better known, and is also open.