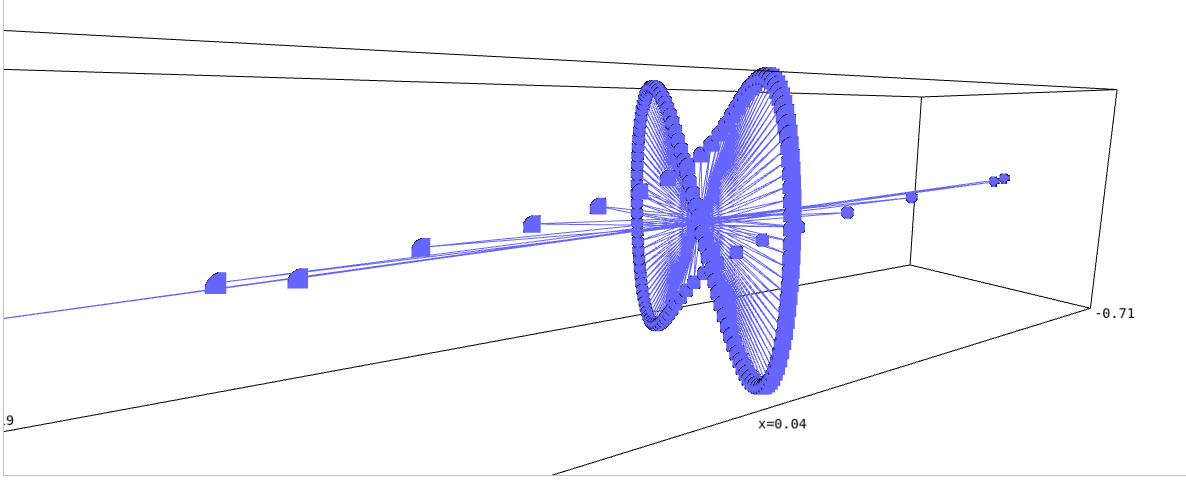
How is this 3d curve called, which looks like a 2d lemniscate but is 3d? (Is there a known parametric equation for it?)

It was generated with this Sagemath - script, where you can zoom in 3d in your browser. The background lies in the following formula of a positive semidefinite matrix, which then is processed with Kernel-PCA to be visualized in 3dim and is related to this question and this question.

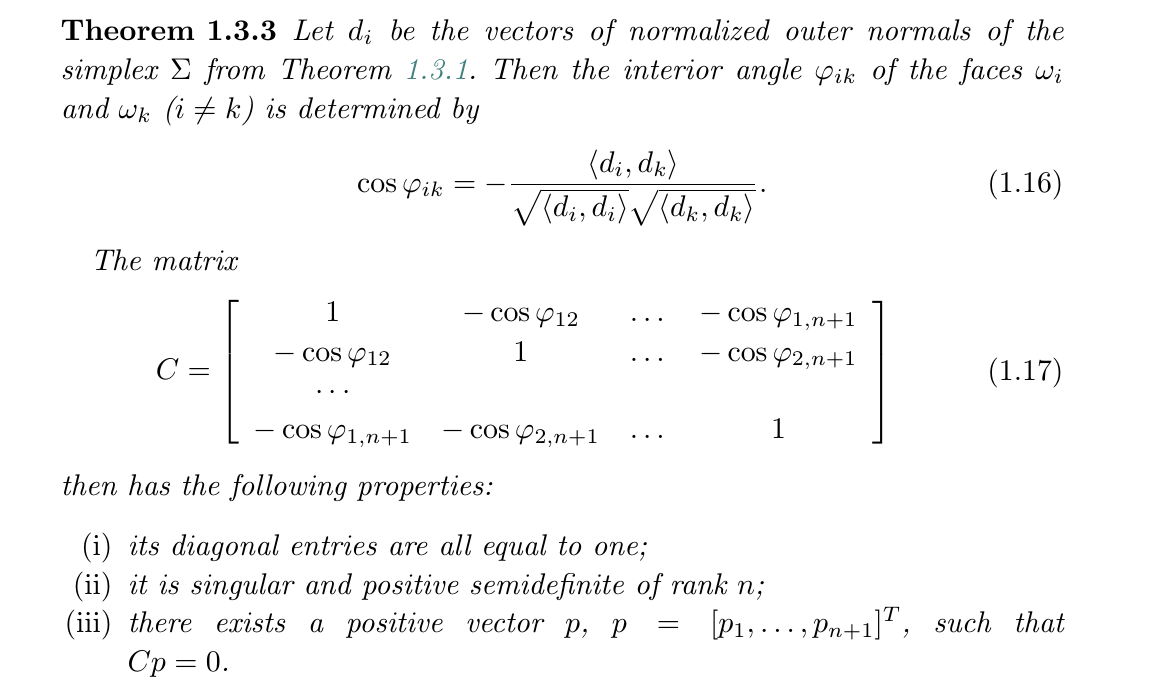
In the book "Matrices and Graphs in Geometry" by Miroslav Fiedler, we have the following generalisation of the sum of angles equals $\pi$ in triangles:

In this case, which we are looking at, we have:

$$G_n^{-1} = (d_1^T,d_2^T,\dots,d_n^T)$$

where

$$G_n = (1/\max(i,j)^2)_{1 \le i,j \le n}$$

is a Gram matrix and $d_{n+1} := \sum_{i=1}^n d_i$. Aftwerwards we treat the entries in the positive semidefinite matrix above as a p.s.d. kernel and thus can visualize its entries $1,\cdots,n+1$ with KernelPCA to get the picture above.

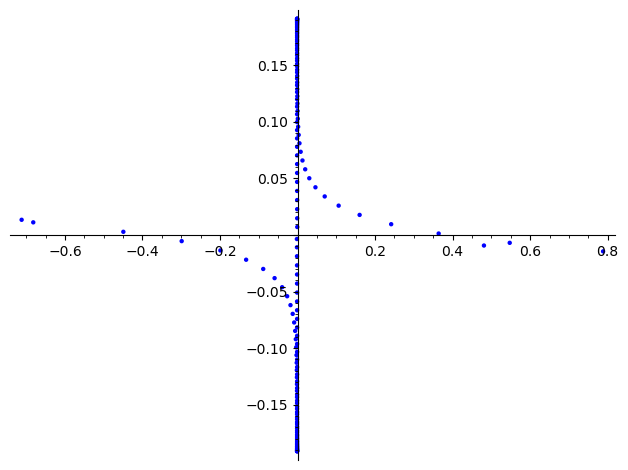
Visualized (with KernelPCA) in 2dim, it looks like this: