(This question is tangentially related to an earlier question I posed.)

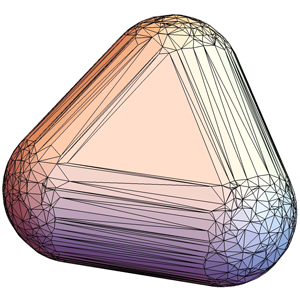
Let $B_1,\ldots,B_k$ be unit-radius balls in $\mathbb{R}^3$, with pairwise disjoint interiors. Let $S$ be the minimal area surface that (a) encloses $B_1,\ldots,B_k$ and (b) is topologically a sphere. Then:

Q. Is it the case that never is $S$ the convex hull of $B_1,\ldots,B_k$?

It may be difficult to specify the exact structure of the minimal surface, but can we at least prove that it always "dents inward"?

The "is topologically a sphere" condition requires the balls to be relatively closely packed. Otherwise $S$ might fracture into several components.

This image from Wrapping juggling balls illustrates the convex hull of three congruent balls: