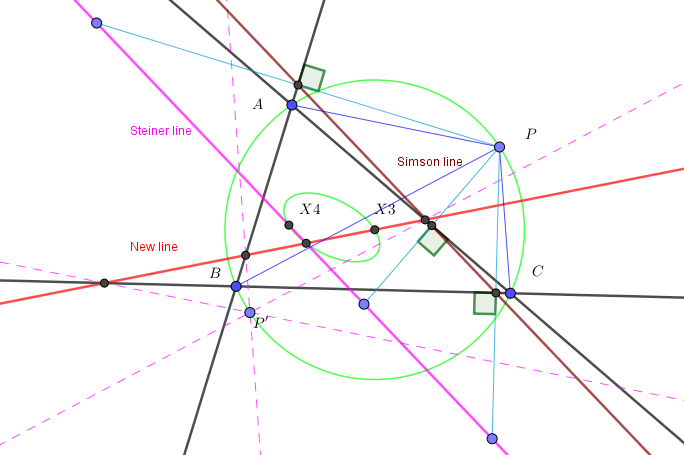
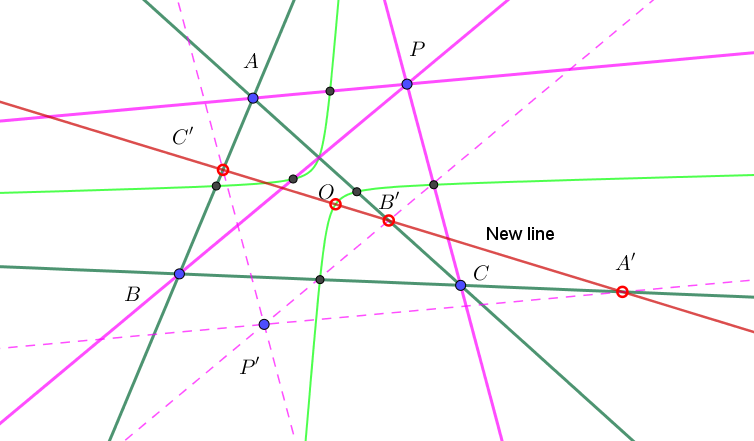
First time, I found a line associated with antipodal points, detail:

Let $ABC$ be a triangle, $(C)$ is circumconic of $ABC$. $P$ and $P'$ are two antipodal points. Construct three lines through $P'$ and parallel to $PA$, $PB$, $PC$ meets $BC$, $CA$, $AB$ respectively at three collinear points, the new line through the center of circumconic.

Question: Is a line associated with antipodal points above known?

Update: But the fact, the result is generalization of the Simson line, I We reformulate as follows:

Let $ABC$ be a triangle, $P$ be a point in the plane, let $C$ is the Nine point conic of $A$, $B$, $C$, $P$. Let $O$ be arbitrary point on $C$, $P'$ is the reflection of $P$ in $O$. Then three lines through $P'$ and parallel to $PA$, $PB$, $PC$ meet three lines $BC$, $CA$, $AB$ respectively at three collinear point.

When $P$ is the Orthorcenter the the line is the Simson line of $P'$

Question again: The generalization of the Simson line above is known?

See also:

• Simson line
• Steiner line