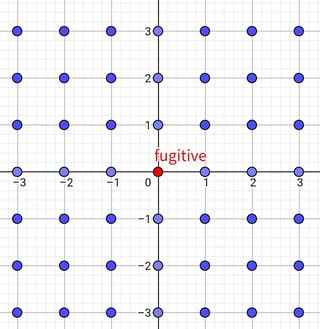
The fugitive is at the origin. They move at a speed of $1$. There's a guard at $(i,j)$ for all $i,j\in \mathbb{Z}$ except the origin. A guard's speed is $\frac{1}{100}$. The fugitive and the guards move simultaneously and continuously. At any moment, all guards move towards the current position of the fugitive, i.e. a guard's trajectory is a pursuit curve. If they're within $\frac{1}{100}$ distance from a guard, the fugitive is caught. The game is played on $\mathbb{R}^2$.

An animation with guards' speed $\frac{1}{4}$ looks something like this (source):

Question: can the fugitive avoid capture forever?

What I know:

1. The fugitive will be caught if they remain in a bounded area.

2. The distance between two guards is strictly decreasing unless the fugitive and the guards remain collinear. But the further away the guards are, the slower that distance decreases.

3. Even if there're only 2 guards, a straight-line dash into the gap between the pair will lead to capture, as long as they're sufficiently far away (see radiodrome).

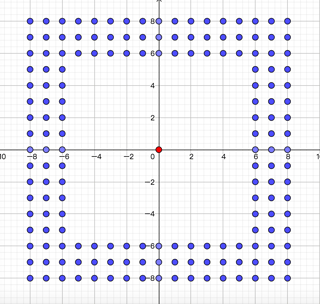
4. The fugitive can escape from arbitrarily large encirclement, provided the wall of guards is not too "thick" (4 or 5 layers are fine), such as this (3 layers):

The shape of the wall doesn't matter (doesn't have to be rectangular).

I asked the question sometime ago on math.stackexchange, where I received the cool animation above, but got no definite answer. I was inspired by a very similar problem here on MO, with additional complication of randomness.