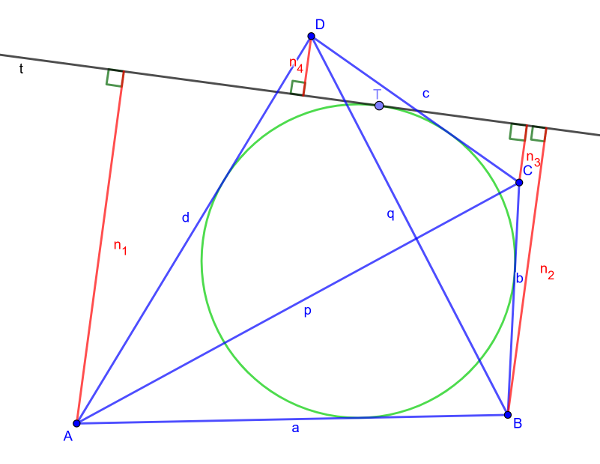
Can you provide a proof for the claim given below? The following claim is inspired by Harcourt's theorem and can be seen as its generalization to quadrilaterals.

Claim. Given bicentric quadrilateral $ABCD$ with area $K$ and semiperimeter $s$ . Denote length of side $AB-a$ ,length of $BC-b$ , length of $CD-c$ length of $DA-d$ , length of diagonal $AC-p$ and length of diagonal $BD-q$. An arbitrary tangent line $t$ is constructed to the incircle of a quadrilateral . Let $n_1$,$n_2$,$n_3$,$n_4$ be a signed distances from the vertices $A$,$B$,$C$,$D$ to tangent line respectively, with a distance being negative if and only if the vertex is on the opposite side of the line from the incenter . Then $\frac{s}{p+q}\left(q(n_1+n_3)+p(n_2+n_4)\right)=2K$ .

GeoGebra applet that demonstrates this claim can be found here.