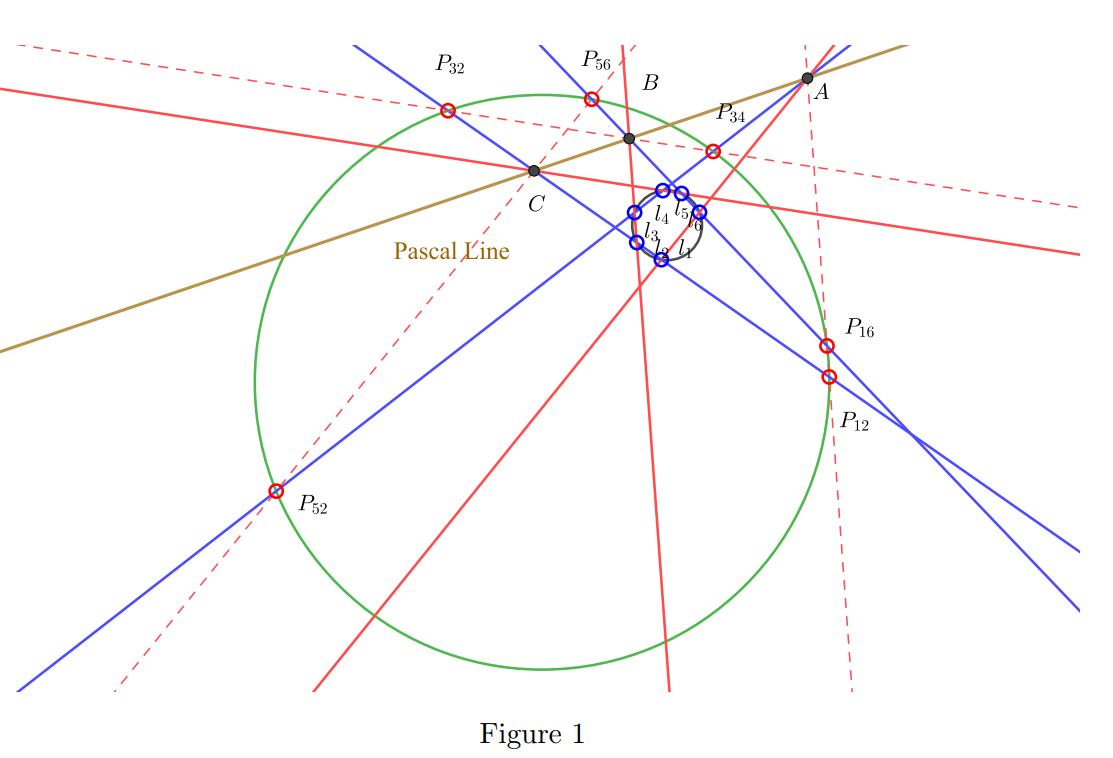
I am looking for a proof or a reference request for a problem as follows:

Problem: Let a cyclic hexagon with sidelines $l_1$, $l_2$, $l_3$, $l_4$, $l_5$, $l_6$ and $l_1 \cap l_4 =A$, $l_3 \cap l_6 = B$, $l_5 \cap l_2 = C$. Let $l’_1$ is the line through $A$ and parallel to $l_3$ meets $l_2, l_6$ at $P_{12}, P_{16}$; $l’_3$ is the line through $B$ and parallel to $l_5$ meets $l_2, l_4$ at $P_{32}, P_{34}$, $l’_5$ is the line through $C$ and parallel to $l_1$ meets $l_4, l_6$ at $P_{54}, P_{56}$. Then show that six points $P_{12}$, $P_{16}$, $P_{32}$, $P_{34}$, $P_{54}$, $P_{56}$ lie on a new circle.