To compute the fundamental group of the complement $S^3 \setminus K$ of a knot, one usually uses the Wirtinger algorithm. Is there a similarly well-established procedure for computing the fundamental group of complements of slice disks in the $4$-ball?

As a partial solution and as an example of what kind of solution would satisfy me, I (and probably also other people) managed to find a cool way of computing ribbon disk complements. Here, a ribbon disk is a smooth disk $R \subset B^4$ in the $4$-ball such that $\partial R \subset S^3$ and the height-function $\Vert \cdot \Vert \rightarrow \mathbb [0, 1]$ restricted to the interior of $R$ has no local maxima.

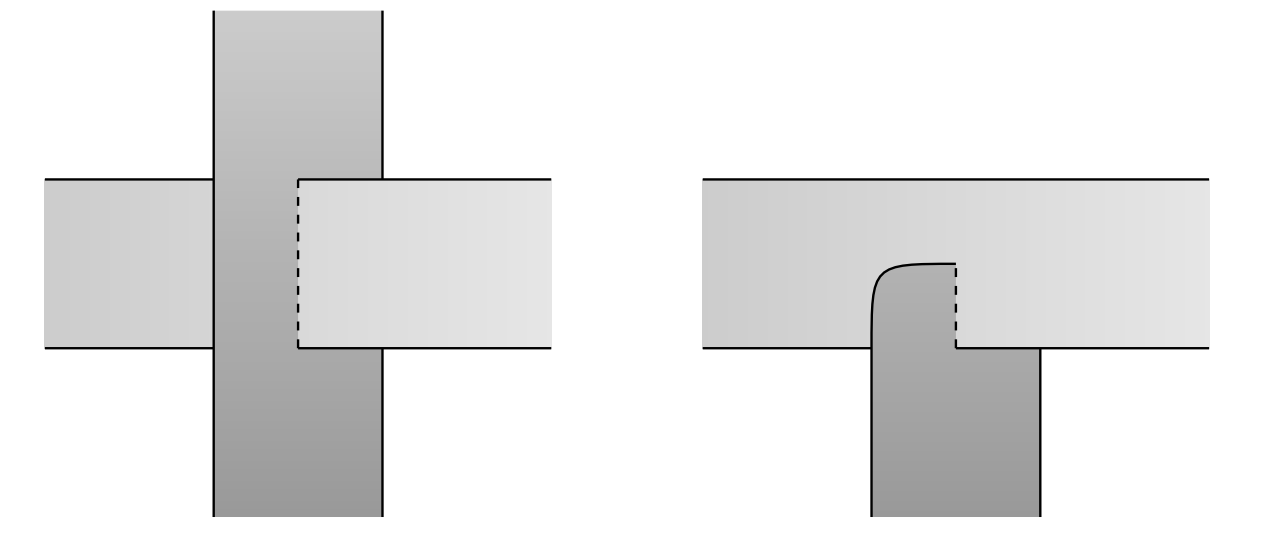
The Wirtinger-like procedure I (and probably also other people) came up with that meets my requirement goes as follows. By a general position argument, after projecting to $S^3$ we can think about $R$ as represented by a "ribbon diagram" $D \subset S^3$, where $D$ is an immersed disk that only has so-called "ribbon singularities": The left-hand singularity in the following picture is a ribbon singularity, but the right-hand singularity is not. 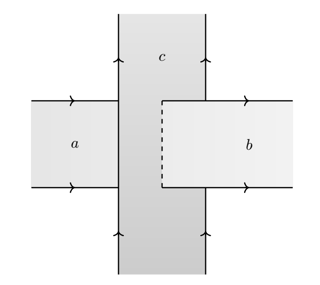These sorts of diagrams will now play the same role as planar knot diagrams play in the usual Wirtinger procedure. Let $S$ be the union of the singular points of $D$. Then an arc of $D$ is a connected component of $D \setminus S$, and a crossing is a connected component of $S$. Orienting the boundary in an arbitrary way, we get a presentation of $\pi_1(B^4 \setminus R)$ by taking the set of arcs of $D$ as generators (interpreted as meridians of $R$ in a very similar way as in the usual setting $K \subset S^3$), and adding the relation $bc=ca$ at every crossing that looks as follows:

The proof that this indeed gives rise to a presentation of $\pi_1(B^4 \setminus R)$ is a van Kampen argument not unlike the one usually used to prove correctness of the Wirtinger algorithm.