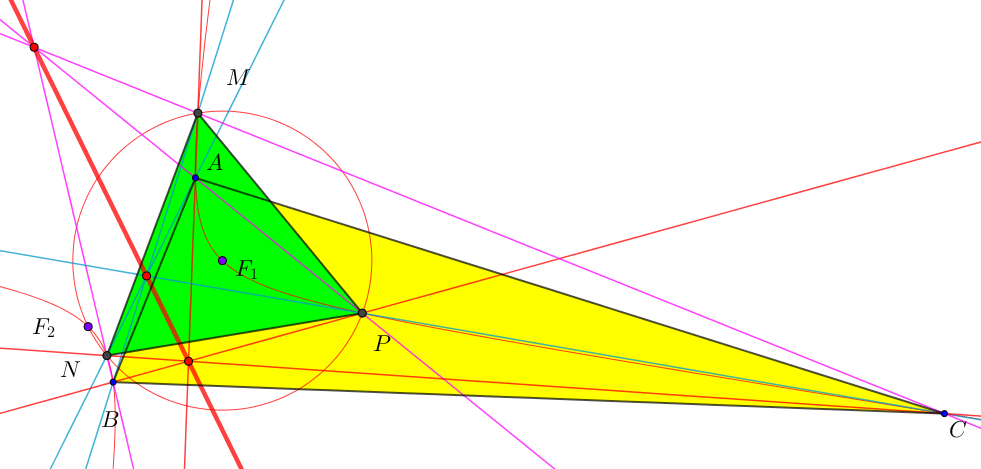
My friend, mister Paul Yiu has sent to me a very new nice equilateral triangle associated with two Fermat points and Kiepert hyperbola of a reference triangle via email since 4 years ago. But I have no proof. This problem is very nice, so I post at here, I hope that have a solution.

1. Let $ABC$ be a triangle with two Fermat points $F_1$, $F_2$. Circles with center $F_1$ radius $F_1F_2$ meets the Kiepert hyperbola again at three points $M$, $N$, $P$.

Then triangle $MNP$ is equilateral and perspective to $ABC$ at triplet points. This mean:

• $MA$, $NB$, $PC$ are concurrent
• $MB$, $NC$, $PA$ are concurrent
• $MC$, $NA$, $PB$ are concurrent

Three points above collinear.

2. Let $ABC$ be a triangle with two Fermat points $F_1$, $F_2$. Circles with center $F_2$ radius $F_2F_1$ meets the Kiepert hyperbola again at three points $M$, $N$, $P$.

Then triangle $MNP$ is equilateral and perspective to $ABC$ at triplet points. This mean:

• $MA$, $NB$, $PC$ are concurrent
• $MB$, $NC$, $PA$ are concurrent
• $MC$, $NA$, $PB$ are concurrent

Three points above collinear

See also:

• Morley's trisector theorem