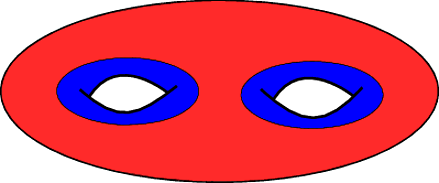
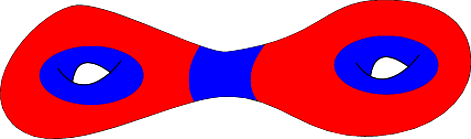
I consider the standard embedding of a compact oriented surface $\Sigma$ (say of genus 2) in the Euclidean space $\mathbb{R}^3$. I have coloured on the picture below the zone of this surface where the curvature is negative (in blue) and where it is positive (in red).
This produces a quite remarkable partition of the surface in the sense that it distinguishes two (homotopy classes) of simple closed curves.
My philosophical question is: to which extend are these simple closed curves characteristic of the embedding? are these curves going to survive deformations through embeddings?
As it is easily seen, one could make this picture more complicated by pinching closed curves and give birth to new non-homotopically trivial zone of negative curvature.
However, this operation seems quite artificial. So would be the operation consisting in adding little bumps within the blue zone to create a little island of red surrounded by a blue zone.
So a more precise question is probably: is there a way of deforming this embedding in $\mathbb{R}^3$ that destroys the blue zone (of the first picture) in an essential way? also, is it possible the fundamental group of the negative curvature locus embeds trivially in the on of the surface after deformation of this initial example?
Thanks!