I was reading about horseshoes and heterclinic bifurcation but my knowledge of dynamical systems is really old fashioned.

as I understand the local stable manifold and the local unstable manifold intersect. I am looking for a differential equation or geometric construction that demonstrates this phenomenon

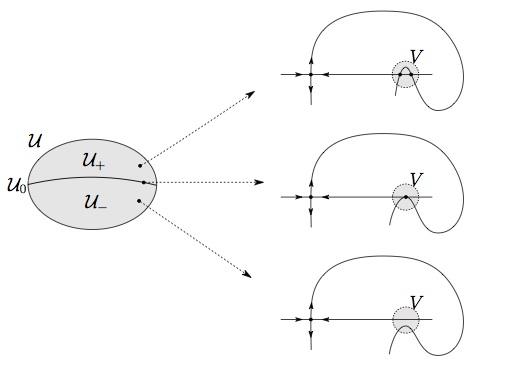
this seems highly improbable. for example the flow defined by

$$ \frac{df }{ dt } = (x^3 + ax +b) f $$

could never exhibit such self-tangent orbits. perhaps I am looking at the wrong place for example?