This is about properties of certain fractals defined by Lindenmayer systems, a.k.a. L-systems. Unlike “classical” fractals like Julia sets or the Mandelbrot set (the name “set” says it all), these fractals are not defined by calculus, but rather geometically, by iterating certain drawing procedures and resizing, which often results in a well-defined limit "curve." Some fractals, e.g. the well-known dragon curve, can be defined by very different L-systems.

I am mainly interested here in fractals where the self-similarity can be seen by decomposing shapes into smaller similar shapes (similar in the strict sense). Which angles can appear between similar shapes? E.g. for the dragon curve, it is easy to see directly from some of the L-system formulae defining it that these angles are $k\pi/8$.

Now I have encountered L-systems featuring angles which do not seem related to one in the formula. E.g. for the following one, the angle between the biggest "hole" and the next-to-biggest one below it (colored blue) is about $70°$. (Note that it is smaller than $72°=2\pi/5$).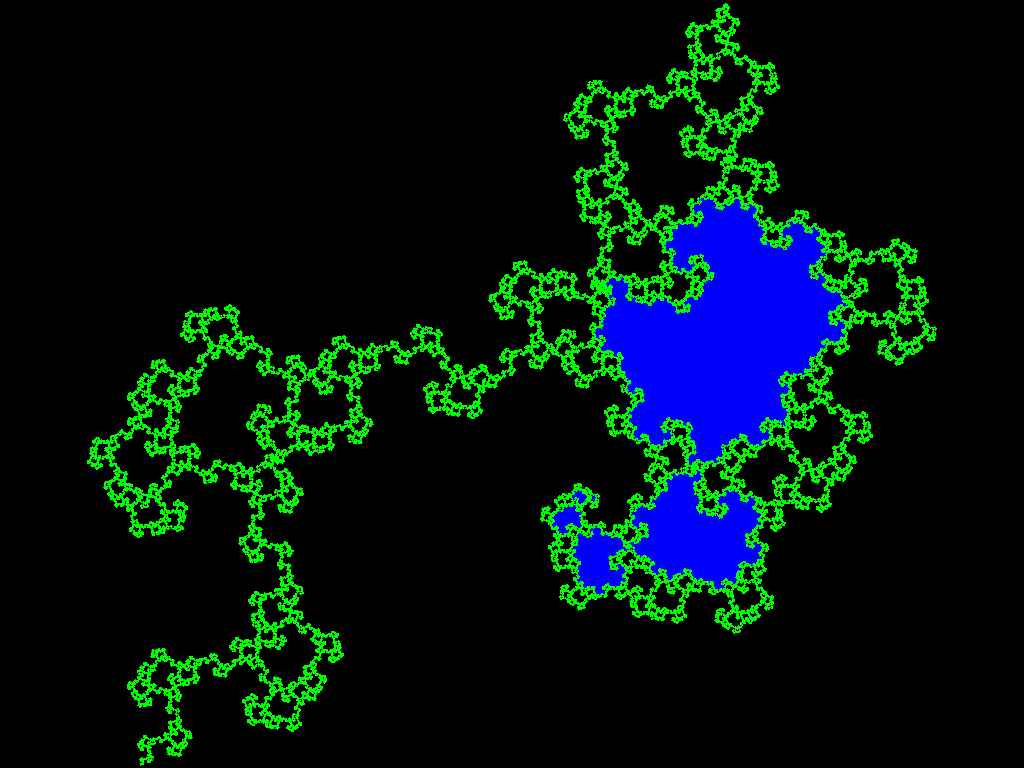
This fractal has been obtained by the formula

Angle 8
Axiom F
F=+!F+!F++!F!+

Or the following one where in the "tail" on the left, subsequent copies of the rectangle-like parts have an angle of about $9°$ to $10°$ between them.
This fractal can be obtained by the formula

Angle 8
Axiom FY 
F=--
X=-XY-F
Y=-X++Y-

It is clear that in both cases above, the formulae do not give any hint about this angle. But my question is:

Can it be shown that the angles occurring in fractals of this kind are always rational?

Similarly, we can wonder about the ratio of sizes among the similar copies contained in the fractal, say the smallest one which is $>1$. Based on some "easy" examples, where this ratio can be derived from the formula, I'd like to ask:

Is this ratio always an algebraic number?